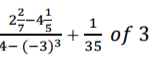KNEC KCSE Mathematics Paper 2 Question Paper / 2015 KCSE Kajiado County Joint Examination
2015 KCSE Kajiado County Joint Examination
Mathematics Paper 2
SECTION I: (50 Marks)
Answer all the questions in this section.
1. � 𝐿𝐿𝐿𝐿𝐿𝐿 6
0.988 𝑥𝑥 9100
3
(4 Marks)
4 marks
The volume Vcm3 of an object is given by V = 2
3
𝜋𝜋3� 1
𝑠𝑠𝑠𝑠2 − 2�
Express c in terms of 𝜋𝜋, r,s and v
4 marks
Simplify the express
5
√7−√5 – 7
√5+√7
3 marks
A boy whose eye level when standing is 1.6m stands infront of a storey building 30m tall. He observes the top of the building at an angle of elevation of 42036’. Find the distance between the boy and the building leaving your answer correct to 4 s.f.
3 marks
Solve for θ in the equation
Sin (2θ – 100) = -0.5 for θ≤0≤3600 (2 Marks)
3 marks
Simplify: 12
1
3 ÷24
32−1
5
2 marks
In the figure below, AB = 3cm, BE = 6cm and DE = 5cm. Find CD.
2 marks
Solve for x in (Log2x)2 + Log28 = Log2x4
3 marks
A steel ball has radius of 15.33mm. Calculate the percentage error in its surface area correct to 2 s.f.
3 marks
Expand �1 + 𝑥𝑥
4
�
5
up to the term x3. Hence evaluate (0.95)5 giving your answer to 4 significant figures.
4 marks
Each month for 30 months, Lemit deposited some money in a saving scheme. In the first month he deposited Sh. 500.
Thereafter he increased his deposits by Sh. 50 every month. Calculate the:
(a) last amount deposited by Lemit (2 Marks)
(b) total amount Lemit had saved in the 30 months (2 Marks)
4 marks
A pond holds 27000 litres of water. How many litres of water would a similar pond hold if its dimensions were double the first one?
4 marks
Position vector of points A and B are a = i + 3j + 5k and b = ui – j + 2k respectively. Find the position vector of point R which divides AB in the ratio 4:-3
3 marks
Juma, Peter and Jane shared KSh. 25,000 as follows: Juma and Peter in the ratio 1:2 and that of Peter to Jane in the ratio 4:1. How much did Peter get?
3 marks
A circle whose centre is (-2,5) has a diameter of 4 units. Find the equation of the circle in its expanded form.
3 marks
Two points on the surface of the earth are A (400N, 300W) and B (200S, 300W). Given that the radius of the earth is 6370km, determination the shortest distance between the two points. (Take 𝜋𝜋 = 22
7 )
3 marks
SECTION II : (50 Marks)
Answer only FIVE questions in this section.
(a) Given A = �
5 1
2 2
�find A-1
(b) Omolo bought 5 bags of maize and 1 bag of beans for Sh. 14000. If Omolo bought 3 bags of maize less and twice
the bags of beans, he would have saved two thousand shillings. If x represents the price of a bag of maize and y
represents he price of a bag of beans.
(i) Form matrix equation to represent the information above. (1 Mark)
(ii) Find the price of a bag of maize and a bag of beans using equation (i) above. (4 Marks)
(c) Find the distance of the point of intersection of the lines 5x + y = 14 and 2y + 2x = 12 from the point (11,-2)
(3 Marks)
10 marks
(a) Complete the table below giving your values correct to 1 decimal place -1800≤x3600. (2 Marks)
x -1800 -1500 -900 -300 00 300 900 1500 1800 2100 2700 3300 3600
y = Sin x -0.5 -0.5 0.5 0.5 -0.5
y = -Sin x 2 -2 2 0
(b) Using the grid provided, draw on the same axis the graphs of y = Sin x and y = -2Sin x (4 Marks)
(c) Use your graph in (b) above to solve the equation Sin x + 2 Sin x = 0 (2 Marks)
(d) What transformation maps y = sin x onto y = -2 Sin x in (b) above. (2 Marks)
10 marks
The figure below shows a shape of a roof with horizontal rectangular ABCD. The ridge EF is also horizontal. The measurements of the roof are AB = 8cm, BC = 5cm, EF = 4.5cm and EA = ED = FB = FC = 3.5cm.
Calculate
(i) the length of the ridge EF above the base ABCD (4 Marks)
(ii) the angle between the face AED and the base ABCD (3 Marks)
(iii) the angle between the face ABFE and the base ABCD (3 Marks)
10 marks
For an in-service course in Mathematics, at least four but not more than nine teachers are to be chosen. The ratio of the number of male teachers to the number of female must be less than 2:1 and there must be more males than females.
If x and y represent the number of male teachers and female respectively.
(a) Write down the inequalities which x and y must satisfy. (4 Marks)
(b) Plot the inequalities in (a) above in the grid provided.
(c) Use your graph in (b) above to find composition of the in-service group of:-
(i) the largest size (1 Mark)
(ii) the smallest size (1 Mark)
10 marks
The table below shows month income tax rates for the year 2003
Monthly taxable income in KSh. Tax rates %
1-9680
9681-18800
18801-27920
27921 – 37040
Over 37040
10
15
20
25
30
The PAYE of Ole Shege in 2003 was Sh. 5079. Ole Shege’s earnings include a basic salary, house allowance of KSh.
120,000, a medical allowance of KSh. 2,880 and commuter allowance of KSH. 340. He was entitled to a monthly tax
relief of KSh. 1056. Calculate:
(i) Ole Shege’s gross tax (1 Mark)
(ii) his basic salary (6 Marks_
(iii)Ole Shege’s net salary if he deducted the following amount from his payslip: (3 Marks)
– NHIF KSh. 320
– Cooperative loan KSh. 2050
10 marks
A bag contains 7 red balls and 5 green balls. A ball is drawn at random three times.
(a) Calculate the probability of drawing three red balls if:
(i) the ball is replaced after each draw. (3 Marks)
(ii) the ball is not replaced after each draw (3 Marks)
(b) Calculate the probability of drawing at least two red balls when the ball is not replaced after each draw.
(4 Marks)
10 marks
(a) The gradient function of a curve is given by 𝑑𝑑𝑑𝑑
𝑑𝑑𝑑𝑑 = 2𝑥𝑥2 – 5 (5 Marks)
Find the equation of the curve, given that y = 3 and x = 2
(c) The velocity, Vm/s of a moving particle after t seconds is given by V = 2t3 + t2 – 1. Find the exact distance covered by the particle in the interval 1≤ t ≤ 3 (5 Marks)
10 marks
Using ruler and a pair of compasses only, construct a triangle ABC such that ∠ABC = 371
2
0, BC = 8cm and AC = 6cm.
Locate a point X in the triangle ABC such that X is equidistant from A, B and C. Measure AX, AB and ∠AXC. (10 Marks)
10 marks