KNEC KCSE Mathematics Paper 1 Question Paper / 2016 Pre KCSE
2016 Pre KCSE
Mathematics Paper 1
SECTION I (50 Marks)
Answer all questions in this section.
Without using a calculator or mathematical table evaluate:
3 marks
Simplify:
3 marks
A straight line passes through the point (-3,-4) and is perpendicular to the line whose
equation is 3x + 2y = 11 and intersects the x=axis and y-axis at points A and B respectively.
Find the length of AB.
3 marks
Evaluate using squares, cubes and reciprocal tables.
4 marks
Given that 
smallest and the largest value of x
4 marks
Makau and Kilonzo live 20km apart. Makau leaves home at 10:00 am and walks to meet
Kilonzo who started walking at 9:30 am to meet Makau. The speed of Makau and Kilonzo
are in the ratio of 3:4. If they met at 11:30 am find their speeds.
3 marks
In the figure below, lines AB and XY are parallel.
If the area of the shaded region is 36 cm2 , find the area of triangle CXY.
3 marks
Given that log a = 0.30 and log b = 0.48 find the value of log b2/c2 .
2 marks
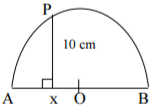
In the figure below O is the centre of the circle diameter AB. <AXP = 90o, AX = 4cm and PX = 10 cm.
Calculate the radius of the semi-circle.
3 marks
The gradient function of a curve that passes through the point (-1,-1) is 2x + 3). Find the
equation of the curve.
3 marks
Evaluate:
3 marks
Estimate the area bounded by the curve y = ½x2 + 1, x = 0, x = 3 and the x-axis using
the mid-ordinate rule. Use three strips.
3 marks
ABCD is a rhombus. The measure of angle ABC is 150o. The diagonals of the rhombus
intersect at E. The shorter diagonal measures 10cm. Calculate the length of the sides of the
rhombus to the nearest integer hence calculate the area of the rhombus.
3 marks
Three police posts are such that Q is on a bearing of 210o and 12 km from P while R is on a
bearing of 150o and 8 km from P.
(a) Using a suitable scale, draw a diagram to represent the above situation. (2 marks)
(b) From the scale drawing determine:
(i) the bearing of Q from R (1 mark)
(ii) the distance of R from Q. (1 mark)
4 marks
A student expands (x – y)2 incorrectly as x2 + y2. Find his percentage error if he used this
incorrect expansion for x = 4 and y = -5. Give your answer correct to 2 d.p.
3 marks
A pulley is made up of two wheels of radii 6 cm and 9 cm respectively and the distance
between their centres is 18 cm.
If a belt passes round the two pulleys, find its length.
4 marks
SECTION II (50 Marks)
Answer any five questions in this section.
A circular lawn is surrounded by a path of uniform width of 7m. The area of the path is
21% that of the lawn.
(a) Calculate the radius of the lawn. (4 marks)
(b) Given further that the path surrounding the lawn is fenced on both sides by barbed wire
on posts at intervals of 10 metres and 11 metres on the inner and outer sides respectively.
Calculate the total number of posts required for the fence. (4 marks)
(c) Calculate the total cost of the posts if one post costs sh 105. (2 marks)
10 marks
A frustum with a regular pentagonal base is such that its top is of side 12cm and bottom is of
side 24cm. If its perpendicular height is 20cm. Calculate:
(a) The length of the slant edge. (5 marks)
(b) The volume of the frustum. (5 marks)
10 marks
Four trucks A, B, C and D take 10 days to transport 42,000 bags of maize to a depot.
However, trucks A and B together take 30 days to transport the same number of bags while
trucks C and D together take 15 days. Truck A carries 1 ½ times the number of bags B
carries and C carries 1 4/5 times as much as D.
(a) Determine the number of bags of maize transported by each truck per day. (5 marks)
(b) All the trucks A, B C and D work together for 5 days, after which truck C and D are withdrawn. A and B work together for another 5 days after which truck A breaks down.
How long does truck B take to complete the rest of the remaining bags? (5 marks)
10 marks
Eunice bought some oranges worth Ksh 45, while Sharon spent the same amount of money
but bought the oranges at a discount of 75 cents per orange.
(a) If Eunice bought an orange at Sh x, write down a simplified expression for the total
number of oranges bought by Eunice and Sharon. (3 marks)
(b) If Sharon bought 2 more oranges than Eunice. Find how much each spent on an orange.
(5 marks)
(c) Find the total number of oranges bought by Eunice and Sharon. (2 marks)
10 marks
(a) The figure shows a velocity time graph of an object which accelerates from rest to a
velocity Vm/s then decelerates to rest in a total time of 54 seconds. If the whole journey
is 810 m,
(i) Find the value of V. (2 marks)
(ii) Find the deceleration given the initial acceleration is 1 2/3 m/s2. (2 marks)
(b) A bus left town x at 10:45 am and travelled towards town Y at an average speed of
60 km/hr. A car left town X at 11:15am on the same day and travelled along the same
road at an average speed of 100 km/hr. The distance between town X and town Y is
500 km.
(i) Determine the time of day when the car overtook the bus. (3 marks)
(ii) Both vehicles continued towards town Y at their original speeds. Find how long the
car had to wait in town Y before the bus arrived. (3 marks)
10 marks
The velocity of a particle t seconds after passing a fixed point O, is given by V = at2 + bt m/s,
where a and b are constants. Given that its velocity is 2 m/s when t = 1 sec and it returns to 0
when t = 4.5 secs, calculate;
(a) The values of a and b. (4 marks)
(b) Hence find;
(i) The values of t when the particle is instantaneously at rest. (2 marks)
(ii) The total distance travelled by the particle during the first 4 seconds. (2 marks)
(iii) The maximum velocity attained by the particle. (2 marks)
10 marks
(a) Complete the table below for the function y = -4 – 6x + 3x2 + 2x3. (3 marks)
| x | -4 | -3 | -2 | -1 | 0 | 1 | 2 |
| y | | | | | | | |
(b) Draw the graph of y = -4 – 6x + 3x2 + 2x3 for values fo x from -4 to 2. (3 marks)
(c) Use your graph to solve.
(i) 2x3 + 3x2 – 4x – 2 = 0 (2 marks)
(ii) 4x3 + 6x2 – 12x – 8 = 0 (2 marks)
10 marks
A parallelogram OACB is such that OA = a, OB = b. D is the mid point of BC OE = hOC
and AE = kAD.
(a) Express the following in terms of a, b, h and k.
(i) OC (1 mark)
(ii) OE (1 mark)
(iii) AD (1 mark)
(iv) AE (1 mark)
(b) Find the values of h and k. (4 marks)
(c) Determine the ratios:
(i) AE : ED (1 mark)
(ii) OE : OC (1 mark)
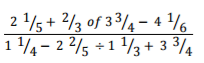








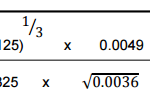



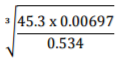

God bless you people
Amen