KNEC KCSE Mathematics Paper 1 – 2014 Nakuru District Mock
2014 Nakuru District Mock
Mathematics Paper 1
SECTION 1 (50 Marks)
ANSWER ALL THE QUESTIONS IN THIS SECTION IN THE SPACES PROVIDED.
Without using mathematical tables or a calculator evaluate.
Leaving the answer as a decimal
3 marks
Two straight highways cross at a right angle at point X. The first highway and the second meet
a straight by-pass at point Y and Z respectively. If YZ is 150km and XZ is 70km. Find XY, to one
decimal place.
3 marks
Factorize 4 PK – 9 + 13 p2 k2
2 marks
The marked price of a revision textbook in a certain bookshop is Kshs, 850. Wilson bought two
dozens of the revision books at a discount of 15%. He sold all of them on the streets making a
profit of 25%. Determine the total sales.
3 marks
The size of each interior angle of a regular polygon is one and a half times the size of the
exterior angle. Find the number of sides of the polygon.
3 marks
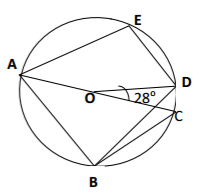
In the figure below AOC is a diameter of a circle centre O. ABDE is a cyclic quadrilateral and
angle COD = 28o.
Determine the size of
(a) Angle AED (2 marks)
(b) Angle CAD (2 marks)
4 marks
Given that θo is an acute angle and tan θo = 1⅓, find without using tables or a calculator
cos (90 – θ )o
2 marks
Solve for y
22y+1 − 6 (2y-1 ) + 1 = 0.
4 marks
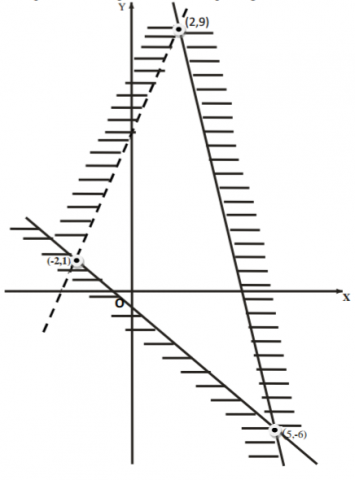
State the inequalities that represent the unshaped region.
3 marks
The position vector of A and B are 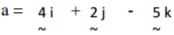
respectively.
Find the magnitude of AB
3 marks
A straight line L1, has its equation as 2x + 3y = 6. Find the equation of a line L2 through
point (-4,5) and parallel to L1, in the form y = mx +c.
3 marks
The figure below show a prism 12cm long. The cross-section is a triangle of sides 4cm, 5cm
and 7cm .
Calculate the surface area of the prism.
3 marks
In a period of two years Kamau paid a simple interest of Kshs.3500 for kshs.5000 borrowed from
Tumaini Bank while Otieno paid a simple interest of Kshs.5600 for Kshs.8000 borrowed from the
same bank. For the same period Kamau paid a simple interest of Kshs.1440 for Kshs.3000
borrowed from Endelea Bank, while Otieno paid a simple interest of Kshs.2400 for Kshs.5000
borrowed from the same Bank.
Determine t he rate of interest charged by each Bank.
4 marks
During a P.E. lesson Sheila stood 50m east of Edna. Both were facing the teacher who was on a
bearing of 045o from Sheila and 065o from Edna. Determine Sheila’s distance from the teacher to
2 decimal places.
4 marks
| 564 | 553 | 566 | 554 | 563 | 563 |
| 657 | 556 | 553 | 554 | 651 | 559 |
Find the quartile deviation.
3 marks
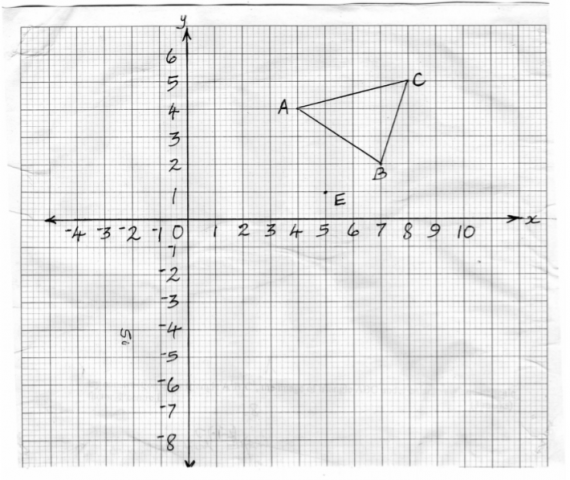
The diagram below shows triangle ABC with vertices A (4, 4), B (7, 2) and C (8, 5)
By construction draw triangle A1B1C1, the image of triangle ABC under enlargement linear scale
factor -2 centre E.
3 marks
SECTION 2 (50 Marks)
ANSWER ANY FIVE QUESTIONS IN THIS SECTION IN THE SPACES PROVIDED.
Four businesswomen decided to buy a building. An agent was selling the building at
Kshs.3,800,000 on behalf of the owner, plus a facilitation fee of 10% of the value of the building
to be paid by the buyers. The agreement was that the buyer would first pay a deposit of 55% of
the total cost and the balance to be paid in one month’s time.
(a) Find,
(i) The amount of deposit paid (3 marks)
(ii) The balance to be paid in one month’s time (2 marks)
(b) The balance was paid in the ratio 1:2:3:5. Calculate:
(i) The money paid by the second highest contributor (2 marks)
(ii) The difference between the money paid by the highest and lowest contributors (3 marks)
10 marks
The figure below shows a solid made up of a conical frustum and a conical top. The dimensions
are as indicated in the figure.
Find
(a) The curved surface area of the conical top (2 marks)
(b) The curved surface area of the frustum (4 marks)
(c) The volume of the solid (4 marks)
10 marks
19.(a) Complete the following table for the equation Y= x3 + 2 x2 – 3x – 3 (2 marks)
| x | -4 | -3 | -2 | -1 | 0 | 1 | 2 | 3 |
| x3 | 64 | _ | _ | -1 | 0 | 1 | 8 | 27 |
| 2x2 | 32 | 18 | 8 | 2 | 0 | 2 | 8 | 18 |
| -3x | _ | 9 | _ | 3 | 0 | -3 | _ | -9 |
| -3 | -3 | -3 | -3 | -3 | -3 | -3 | -3 | -3 |
| y | _ | -3 | 3 | _ | _ | -3 | 7 | _ |
(b) On the grid provided draw the graph of y= x3 + 2 x2 – 3x – 3 for -4 ≤ ≤ 3. Use 2cm to
represent I unit on the x-axis and 1cm to represent 5 units on the Y-axis. (3 marks)
(c) (i) Use the graph to estimate the roots of the equations x3 + 2 x2 – 3x – 3 = 0 (2 marks)
(ii) By drawing a suitable line use the graph in (b) above to obtain the roots of the equation −x3 − 2x2 + 5x + 8 = 0 (3 marks)
10 marks
Sally bought some mangoes worth Kshs.60, while Peris spent kshs.60, but bought them at a
discount of 50cents per mango.
(a) If Sally bought a mango at sh. X. write down a simplified expression for the total number of
mangoes bought by
(i) Sally (1 mark)
(ii) Peris (1 mark)
(b) If Peris bought 3 more mangoes than Sally. Find how much each spent on a Mango (to the
nearest cents) (5 marks)
(c) Find the total number of mangoes bought by Sally and Peris (to the nearest whole number)
(3 marks)
10 marks
A plastic model of a tank, open at the top is in the shape of a cylinder. The internal radius of its
base is r cm and its internal height is h cm. The total internal surface area of the tank is
1386 cm2.
(a) Write an expression for the total internal surface area of the tank (1 mark)
(b) Express in terms of r
(i) The internal height of the tank (2 marks)
(ii) The internal volume of the tank ( 1mark)
(c) Determine
(i) The value of r for which the internal volume, V is maximum (4 marks)
(ii) The maximum internal volume of the tank (2 marks)
10 marks
A plane P is 16500 km on a bearing of N 30o E from an international airport. Another plane Q is
9,900km on a bearing of S 60oE from plane P. Plane R is 23,000km due south of the airport.
(a) Using a scale of 1cm to represent 3000km, show the relative positions of P, Q R and the
airport. (3 marks)
(b) Find the distance and bearing of R from Q (3 marks)
(c) If Q and P are both travelling at 4000km/hr towards the airport. Calculate the difference in
the time taken to reach the airport by the two planes, to the nearest hour. ( 4 marks)
10 marks
The frequency distribution table below shows the K.C.P.E. marks obtained by peoples in a certain
school.
| Marks | number of peoples |
| 200 ≤ x ≤ 220 220 ≤ x ≤ 240 240 ≤ x ≤ 280 280 ≤ x ≤ 320 320 ≤ x ≤ 340 |
6 14 12 8 5 |
(a) Estimate the mean marks of the peoples (4 marks)
(b)(i) On the grid provided, draw a histogram to represent the information above (3 marks)
(c) )i) State group in which the median mark lies ( 1mark)
(ii) A vertical line drawn through the median mark divides the total area of the histogram into two
equal parts. Using this information or otherwise, estimate the median mark (2 marks)
10 marks
Triangle ABC has the vertices A (3, 1), B (2, 2) and C (3, 4).
(a) On the grid provided draw triangle ABC and its image A1B1C1 under a rotation of negative
quarter turn about the point (0,0) (3 marks)
(b) (i) Draw triangle A11B11C11 the image of ∆ A1B1C1 under a reflection in the line y = -x 2 marks)
(ii) Describe fully the transformation that maps ∆A11B11C11 onto ∆ABC (2 marks)
(c) (i) On the same axes draw triangle A111B111C111 the image of ∆ A11B11C111 under a translation
given by translation Vector −6 1 (2 marks)
(iii) State the co ordinates of ∆A111B111C111(1 mark)
10 marks