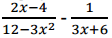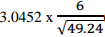KNEC KCSE Mathematics Paper 1 Question Paper / 2016 KASSU JET JOINT EXAMINATION

Evaluate

Solve for x in sin(x– 15) – cos x(x + 5) =0

The LCM of two numbers is 328,600 and the GCD is 20. If one of the numbers is 1240, use
prime factorization method, find the other number.

A sperical solid lead of diameter 12cm weighs 6.4kg. How much would a similar solid of a
diameter 10cm weigh?

Without using a calculator or mathematical tables evaluate,

On arrival to Kenya a Canadian tourist exchanged his Canadian dollars for Ksh 199 690.
Given that the currency exchange rate was 1 Canadian dollar = Ksh 52.55 and that the bank
charged him 5% commission, find the number of dollars he exchanged.

By using completing square method, solve for x in 4x² – 3x – 6 = 0

Simplify the following.

The matrix  maps a triangle ABC onto a straight line. Determine the possible
values of x.

Using the tables of squares, square roots and reciprocal 

Find the percentage error in the quotient in 9.16𝑐𝑐𝑐𝑐 ÷ 2.0

The position vectors 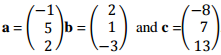. Find the scalars S and T such that

Sa + Tb = c

The following data represents the enrolment of students in 12 colleges
564 553 566 554 563 563
657 556 553 554 651 559
Calculate the quartile deviation.

The density of a sphere of diameter p cm is 2.68 g/cm³ and that of another sphere is diameter
Q cm is 14.23 g/cm³. Determine the volume of sphere Q that would have the same mass as
80cm³‍

Solve and represent the integral values of the linear inequalities given below on a number line.

Find the equation of the normal to the curve y = x³ – 2x² + 3x – 1 at the point ( 2,5)

A straight line L₁ has its x-intercept and y-intercept as -6 and 4 respectively.
a) Write its equation in the form ax +by +c =0 where a, b, and c are integers (3marks)
b) Another line L₂ which is parallel to L₁ in (a) above passes through (2,3k) and (-k,8). Find
the value of k. (2marks)
c) Find the equation of the perpendicular bisector to the line L₁ (3marks)
d) Calculate the angle which L₁ makes with the x-axis (2marks)

A man spent 1/9 of his salary on food and 1/4 of the remainder n electricity and water bills. He
paid fees with 20% of his salary and invested 16% of what was left into a business. After
taking a game drive on which he spent Ksh 2000, he saved Ksh 5350. Calculate:
(a) His total monthly earnings. (4 marks)
(b) How much he spent on fees. (2 marks)
(c) How much he invested. (2 marks)
(d) The percentage of the salary saved. (2 marks)

Every Sunday Alex drives a distance of 80km on a bearing of 074^o to pick up his brother
John to go to church. The church is 75km from John’s house on a bearing of S50^oE. After church they drive a distance of 100km on a bearing of 260^o to check on their father before Alex
drives to John’s home to drop him off then proceeds to his house.
(a) Using a scale of 1cm to represent 10km, show the relative positions of these places.
(4 marks)
(b) Use your diagram to determine:
(i) the true bearing of Alex’s home from their father’s house. (1 mark)
(ii) the compass bearing of the father’s home from John’s home. (1 mark)
(iii) the distance between John’s home and the father’s home. (2 marks)
(iv) the total distance Alex travels every Sunday. (2 marks)

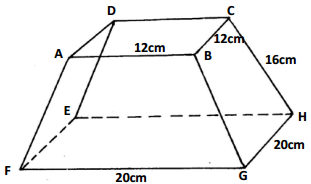
The figure below shows solid frustum of a pyramid with a square top of side 12cm and a square base
of side 20cm. The slant edge of the frustum is 16cm.

a) Calculate the total surface area of the frustum (4marks)
b) Calculate the volume of the solid frustum. (4marks)
c) Calculate the angle between the planes BCHG and the base EFGH. (2marks)

(a) A radio station tower was built in two sections. From a point 870m from the base of the
tower, the angle of elevation of the top of the first section is 250 and the angle of elevation of the
top of the second section is 40^o. What is the height of the top section of the tower? (5marks)
(b)Two vertical poles on horizontal ground are 60m apart. The shorter pole is 3m high. The angle
of depression of the top of the shorter pole from the top of the longer pole is 20^o. Using scale
drawing, find the length of the longer pole. (5 marks)

Coast bus left Nairobi at 8.00a.m. and traveled towards Mombasa at an average speed of
80km/hr. at 8.30am, Lamu bus left Mombasa towards Nairobi at an average speed of 120km/h.
Given that the distance between Nairobi and Mombasa is 400km; determine:
(i) The time Lamu Bus arrived in Nairobi. (2marks)
(ii) The time the two buses met. (4marks)
(iii) The distance from Nairobi to the point where the buses met. (2marks)
(iv) How far Coast Bus is from Mombasa when Lamu bus arrives in Nairobi. (2marks)

Triangle PQR is inscribed in the circle. PQ = 7.8cm, PR = 6.6cm and QR = 5.9cm.

Find;
(a) size of angle QPR (3 Marks)
(b) the radius of the circle. (3 Marks)
(c) the area of the shaded region. (4Marks)

(a) Find the stationary points of the curve to (1 d.p) (6 marks)

(b) Find the x and y intercepts of the curve above. (2 marks)
(c) Sketch the curve. (2 marks)