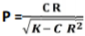KNEC KCSE Mathematics Paper 2 – 2014 Nakuru District Mock
2014 Nakuru District Mock
Mathematics Paper 2
SECTION I (50 Marks)
Answer all questions in this section in the spaces provided.
Use logarithms to evaluate
4 marks
A bath tub has two inlet pipes P1 and P2 and an outlet pipe P3. Pipe P1 can fill an empty bath
tub in 15 minutes while pipes P1 and P2 , when opened at the same time can fill the same empty bath
tub in 6 minutes. P3 can empty the tub in 12 minutes. Find the fraction of the tub filled if P2 and P3
are opened for 25 minutes.
3 marks
To obtain the estimate value of 1056 ÷ 22, the numbers were first rounded off to the
nearest ten. Calculate the percentage error arising from this rounding – off.
3 marks
Make R the subject of the formular
3 marks
Without using mathematical tables or a calculator simplify
2/3 log 27 – log 5 + log 45
3 marks
(a) Expand and simplify (1 + ¼x )6 ( 2 marks)
(b) Use t he expansion in (a) above up to the fourth term to estimate the value of (1.025)6 ( 2 marks)
4 marks
In the diagram below ( not drawn to scale,) PR and QT are chords of a circle intersecting at
S. OT is a tangent to the circle at T. Chord QP produced meets the tangent at O.
(a) Given that O T = 10.5 cm and O P = 8 cm. Calculate the length of P Q (2 marks)
(b)Hence determine, to 1 decimal place the length of TR if PS = 4.3 and TS = 2.3 cm. (2 marks)
4 marks
A mixture P contains sorghum and millet in the ration 2: 3. Another mixture Q contains
sorghum and millet in the ration 3:1. 15kg of P is mixed with 24kg of Q, determine the ratio
of sorghum and millet in the new mixture.
3 marks
Rationalize the denominator leaving your answer in the form a + b where a, b and c are
constants.
2 marks
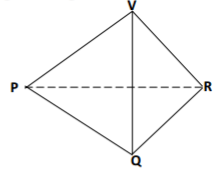
The figure below is a regular tetrahedron VPQR with edges of length 6cm.
Calculate the angle between the planes VQR and PQR .
3 marks
Solve for x: tan2 x – 2 tan x = 3 for the interval 0 < x < 180o
3 marks
A quantity P varies partly as the square root of Q and partly as the inverse of Q. Given that
P = 14.5 when q = 4 and P = 17 when Q = 9 determine the equation connecting P and Q.
4 marks
A car travelling at 94 km/hr is 5 m behind a truck travelling at 80 km/hr. If the truck is 13 m
long and the car is 3 m long, deter mine the time taken in seconds for the car to completely
overtake the truck.
3 marks
The figure below shows a sketch of a graph of a quadratic function
Y = 3 c (x – 2) (x – 5).
Find the value of C.
2 marks
Given that 
Determine the value of x, hence state the two possible matrices.
3 marks
The equation of a circle is given by 5x2 + 20 x + 5y2 – 25 = O. Find the radius and the centre
of the circle.
3 marks
SECTION II (50 Marks)
Answer any five questions in this section in the spaces provided.
The table below shows the income tax rates in 2012.
| Income (K £ per annum) | Tax rate (Kshs. Per K £) |
| 1-5808 5809-11280 11281 – 16752 16753 – 22224 22225 – 27696 Over 27896 |
2 3 4 5 6 6.5 |
In June 2012 Mrs Sudi earned the following per month: a basic salary of Kshs.23530, a house
allowance of Kshs.8000, a medical allowance of Kshs.2844 and a commuter allowance of
Kshs.2031.
She was entitled to a personal relief of Kshs.1056 per month.
(a) Calculate:
(i) Her taxable income in K£ per annum (1 mark)
(ii) The net tax paid by Mrs. Sudi in Kshs per annum (5 marks)
(b) In July 2012, Mrs. Sudi’s Basic salary was increased in the ration 11:10 and received a hardship
allowance that is 30% of her basic salary. Find the additional net tax per annum as a
percentage (significant figures,) of the net tax obtained in (a)(ii) above ( to 4) (sig.fig) (4 marks)
10 marks
In a mathematics test, the probability of 3 students, Kamau, Otieno and Mwala passing are
⅔,¾ and
(a) Draw a tree diagram to represent this information (3 marks)
(b) Use the tree diagram to find the probability that:
(i) All the three students will fail (2 marks)
(ii) At least two students will pass. (3 marks)
(iii) Only one student will pass (2 marks)
10 marks
The position of two towns A and B are given to the nearest degree as A (40oN, 110oE) and
B (50oN, 70oW).
(a) Find the shortest distance between the two towns in kilometers. (Take the radius of
the earth as 6370 km). (3 marks)
(b) An aircraft flew through the shortest route from town A to town B and then proceeded
to town C, 6000 nm due south of B at an average speed of 850 km/h.
(i) Find the position of C
(ii) Find the time taken by the aircraft to fly from town A to town C. ( 2 marks)
(iii) Determine the local time at A when the local time at C is 5.30 p.m. (2 marks)
10 marks
In triangle OPQ below, R and S are points on OQ and PQ respectively, such that the ratio
PS: SQ= 2:1 and OR = ½ OQ. T is a point on OS such that OT: TS= 3:2.
Given that 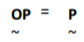
(a) Express the following vectors in terms of 
(i) 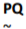
(ii) 
(iii) 
(iv) 
(b) Show that P, T and R are collinear (4 marks)
(c) Determine the ratio PT:TR (1 mark)
10 marks
(a) Using a pair of compasses and a ruler only. Construct triangle ABC such that AB=7cm
BC= 6cm and angle ABC = 60o
. Measure AC (3marks)
b) On the same side of AB as C. (i) Determine the locus of points P such that angle APB =60o
(2marks)
(ii) Construct the locus of R such that AR= 4.5cm (1 mark)
(iii) By shading the unwanted region identify the region T such that AR 4.5. Angle APB 60o and
angle ACB angle BCA . (4 marks)
10 marks
Laptech company is considering installing two types of machines, Type A and type B, for
assembly of spare parts of laptops. Type A machine can assemble 5 spare parts per hour
while type B machine can assemble 3 spare parts per hour. Type A machine requires 11
operators while type B machine requires 9 operators. The number of type B machines
must be more than the number of type A machines. The total number of spare parts
assembled per hour must be at least 30 and the number of operators should not exceed 100.
There should be at least 3 type A machines and at least 4 type B machines.
(a) Taking x to be the number of type A machines and y to be the number of type B machines.
Write down in terms of x and y the linear inequalities representing the information above
(4 marks)
(b) On the grid provided draw the inequalities and shade the unwanted regions. (4 marks)
(c) If the company makes a profit of shs.6 per hour on type A machines and shs.2 per hour on
type B machines, Use the graph in (b) above to determine the number of machines of each
type that should be installed to maximize the profit. (2 marks)
10 marks
A Manson lays bricks in the erecting of a perimeter wall. In consecutive days, he increased
the number of bricks laid by an equal number. On the third day he laid 23 bricks, while on
the seventh day he laid 35 bricks.
(a) Calculate
(i) The number of bricks he laid on the first day (2 marks)
(ii) The constant increase of the number of bricks laid daily (2 marks)
(iii) The number of bricks laid on the eleventh day (2 marks)
(b) If he laid 80 bricks on the last day, find the total number of bricks laid (4 marks)
10 marks
The relationship between the pressure P of a fixed mass of a gas and its volume V at a
constant temperature, is known to be of the form P= k/V where K is a constant. The
table below shows the experimental results for pressure and corresponding values of
volume.
| Pressure (N/cm2) |
1.1 | 1.8 | 2.2 | 2.6 | 3.4 |
| Volume (Litres) |
3.03 | 2.12 | 1.65 | 1.4 | 1.07 |
(a) Using the grid provided, plot the graph of P against 1/V ( 5 marks)
(b) From the graph estimate the value of K (3 marks)
(c) Determine the volume of the gas when the pressure is 3.1 N/cm2(2 marks)
10 marks