KNEC KCSE Mathematics Paper 2 Question Paper / 2016 KCSE KAMDARA JET Examination
2016 KCSE KAMDARA JET Examination
Mathematics Paper 2
SECTION I (50 Marks)
Answer all the questions in this section in the spaces provided below each question.
Using an assumed mean of 50 , calculate the standard deviation of the marks obtained in a test recorded as follows: 50, 52, 45, 40, 55, 51 56, 48, 55, 60
2 marks
Make x the subject of the formula
3 marks
Find the value of x in the equation
Log3 X – 4logX3 = -3
4 marks
a) Expand the binomial (2 – ¼ x)5(2 marks)
b) Using the first 4 terms of the binomial above solve for 1.755 (2 marks)
4 marks
a) Find the inverse of the matrix 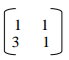
b) Hence determine the point of intersection of the lines (2 marks)
x + y = 7
3x + y = 15
3 marks
Rationalise the denominator and simplify the answer completely.
3 marks
Solve for x in the trigonometric equation 4cos2x + 4sin2 x = 16sin2xcos2x in 0o ≤x≤360o
5 marks
The mass of a cylinder of a small material varies jointly as the square of the radius and as the
height. If the radius is increased by 20% and the height by 10%. Find the percentage increase in
mass. (3 marks)
3 marks
Given that the dimensions of a rectangle are 20.0cm and 25.0. Find the percentage error in calculating the area.
3 marks
Maina bought a new laptop on hire purchase. The cash value of the laptop was Ksh. 56,000. He paid a deposit of Ksh. 14,000 followed by 24 equal monthly installments of Ksh. 3500 each. Calculate the
monthly rate at which the compound interest was charged.
3 marks
Find the equation of tangent to a curve x2 = 4y+1 at the point (2, 0.75)
3 marks
Object A of area 12cm2 is mapped onto its image B of area 72cm2 by a transformation. Whose matrix is given by 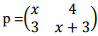
3 marks
In the figure below, AB is a tangent, meeting chord CDE at B. AD = 5cm, CD = 4cm, DF = 2cm, EB =
7.5cm and DE = x cm.
Determine:
(a) The value of x (1mark)
(b) The length of AB. (2 marks
3 marks
A ship covers 60km on a bearing of 230o. If then it changes course and heads due west for
80km, determine its direct distance from the starting point.
3 marks
Find the centre and the radius of the circle whose equation is x2 + y2 – 7x + 6 + 11y = 0
3 marks
The 2nd, 4th and 7th terms of A.P are the first 3 consecutive terms of a G.P. Find:
(a) The common ratio (2Marks)
(b) The sum of the first eight terms of the G.P if the common difference of the A.P is 2.
(2Marks)
4 marks
SECTION II (50 Marks)
Answer ONLY FIVE questions in this section in the spaces provided.
In the figure above, M divides line OB in the ratio 1:2 and N divides 

intersect at X. Given 
a) Find in terms of a and b :
(i) 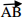
(ii)
(iii)
b) If 

(i) Express 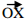
Hence find the value of h and k (4 marks)
c) Find the ratio of 
(i) Express OX in two ways. ( 2 marks)
10 marks
The figure below shows a right pyramid with a rectangular base. The length of the rectangular base is
15cm and the width is 8cm. The slant edges are all equal to 20cm.
Calculate
a) The volume of the pyramid. (3 marks)
b) The angle VAB makes with ABCD (3 marks)
c) The angle plane XBD makes with VBD given that point X lies on VA such that VX: XA = 2: 3
(4 marks)
10 marks
The number x is chosen at random from the set (0,3,6,9) and the number y is chosen at random
from the set (0,2,4,6,8). Calculate the probability of each of the following separate events.
(i) x > 6 (1 mark)
(ii) x + y = 11 (2 marks)
(iii) x > y (3 marks)
(iv) xy = 0 (2 marks)
(v) 10x + y < 34 (2 marks)
10 marks
P and Q are two points on the same parallel of latitude 66o 251, whose longitudes differ by
120o. Calculate in kilometres. Radius of the earth =6370.
a) The radius of the parallel of latitude where P and Q lie. (2 marks)
b) The distance of P and Q measured along the parallel of latitude. (2 marks)
c) (i) find the length of the straight line joining PQ (2 marks)
(ii) Find the distance between P and Q along the same latitude in nautical miles. (2 marks)
(iii)If an aircraft took 30min to fly from P to Q, Calculate its speed in knots. (2 marks)
10 marks
a) Use the trapezium rule to estimate the area between the curve y = 3x2 + 1, lines x=1 and x=3
and x-axis. Use five ordinates. (5 marks)
b) Using integration method find the exact area under a curve y=3x2 + 1 (3 marks)
d) Find the percentage error in estimating the area. (2 marks)
10 marks
The table below shows the rate at which income tax is charged for all income earned in a month
in 2015.
| Taxable Income p.m (Kenya pound) 1 -236 237 -472 473 -708 709 – 944 945 and over |
Rate in % per Kenya pound 10% 15% 20% 25% 30% |
A total of Ksh. 14,500 is deducted from Mrs. Momanyi monthly salary .She is entitled to a house
allowance of Ksh. 8,000 a person relief of Ksh. 1064 month and Monthly insurance relief at the rate
of 15% of the premium paid. Every month she pays the following.
(i) Electricity bill shs.780
(ii) Water bill shs. 560
(iii) Co-operative shares shs. 1200
(iv) Loan repayment Ksh. 5000
(v) Monthly insurance premiums of Ksh 1260
(a) Calculate her P.A.Y.E (2Marks)
(b)Calculate her monthly taxable income . (6Marks)
(c) Calculate her basic salary per month (2Marks)
10 marks
Mr. Wanyama wishes to take student from wonderful mixed secondary school for a tour. The total
number of pupils to be taken should not exceed 60. Each girl must contribute sh.10,000 and each boy
sh.15,000 and money to be contributed must not exceed sh.120,000. If this trip is to be successful the number of boys must conditionally be greater than girls.
a) Write down five inequalities to represent this information taking the number of boys and girls to be
x and y respectively. (4 marks)
b) Represent the above information on the graph paper below. (4 marks)
c) What is the optimum number of boys and girls to be taken in order to be minimise cost. (2 mark)
10 marks
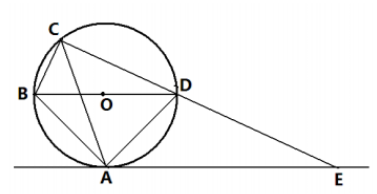
In the figure below, line BD is the diameter of the circle, centre O and AE is a tangent.
Angle CBA = 110o and angle BAC =26o
Find the following angles, giving reasons for each answer.
a) ∠ABD (3marks)
b) ∠DAE (1mk)
c) ∠AED (3marks)
d) ∠AOD (3marks)
10 marks