KNEC KCSE Past Papers 2019 Mathematics Alt A Paper 2 (122/2)

KNEC KCSE Past Papers 2019 Mathematics Alt A Paper 2 (122/2)

Kenya Certificate of Secondary Education

2019 Mathematics Paper 2

Section I (50 marks)

Answer all the questions in this section.

1. Simplify √5+3∕ √5-2 . Give the answer in the form a + b∕c where a, b and c are integers. (2 marks)

2. Two types of flour, X and Y, cost Ksh 60 and Ksh 72 per kilogram respectively.

The two types are mixed such that the cost of a kilogram of the mixture is Ksh 70. Calculate the ratio X:Y of the mixture.(3 marks)

3. A quantity P varies inversely as the square of another quantity L.

When P = 0.625, L = 4. Determine P when L — 0.2. (3 marks)

4. An arc of a circle subtends an angle of 150° at the circumference of the circle.

Calculate the angle subtended by the same arc at the centre of the circle. (2 marks)

5. Solve the equations:

x + 3y = 13
x² + 3y² = 43 (4 marks)

6. A bag contains 6 red counters and 4 blue counters.

Two counters are picked from the bag at random, without replacement.

(a) Represent the events using a tree diagram. (1 mark)

(b) Find the probability that the two counters picked are of the same colour. (2 marks)

7. Find the coordinates of the turning point of the curve y=x²-14x+10 (3 marks)

8. OAB is a Sector Of a circle of radius r cm. Angle AOB = 60°.

Find, in its simplest form, an expression in terms of r and z for the perimeter of the sector.(2 marks)

 

9. In a mathematics test, the scores obtained by 30 students were rccordcd as shown in the toblc below.

 

Score (x)	59	61	65	K	71	72	73	75
No. of students	2	3	5	6	7	4	2	1

The score K with a frequency of 6 is not given.

Given that ⅀fd∕⅀f = — 1.2 where d —— x – 69, and using an assumed mean of 69, determine score K.

10. Determine the amplitude and the period of the function y = 3 sin(2x + 40°). (4 marks)

11. The figure ABCDEFGH represents a box.

 

The top lid of the box is opened such that the height OT is 35cm. Calculate the:

(a ) angle the top lid makes with the plane FGHE;

(b) length BE, correct to 2 decimal places.

12. The table below’ shows income tax rates in a certain year.

 

Monthly Income in ksh	Tax rate  in each shilling (%)
0  – 10164	10
10165 – 19740	15
19741 – 29316	20
29317 – 38892	25
38893 and above	30

In that year,mawira earned a salary of 41000 per month.calculate mawira’s income tax per month given that a monthly tax relief of ksh 1162 was allowed (3 marks)

13. The position vectors of points A,B and C are OA =[3/4],OB = [1/2] and OC =[7/-1] show that A,B and C are collinear (3 marks)

14. The vertices of a triangle PQR are P(-3, 2), Q(0, — 1) and R(2, — 1). A transformation matrix maps triangle PQR onto triangle P’Q’ R’ whose vertices are P'(—7, 2), Q'(2, — 1) and R’(4, – 1).

Find M°’, the transformation that maps P’Q’R’ onto PQR.(4 marks)

15. Solve for x in log(7x—3) + 2 log 5 = 2 + log(z + 3).(4 marks)

16. The length of a shadow’ of a mast was measured at intervals of 1 hour and recorded as shown in the table below.

 

Time( hr)	0	1	2	3	4	5
Length( m)	18.7	8.7	5.0	2.9	1.3	0

(a) On the grid prov’ided, draw the graph of length against time. (2 marks)

 

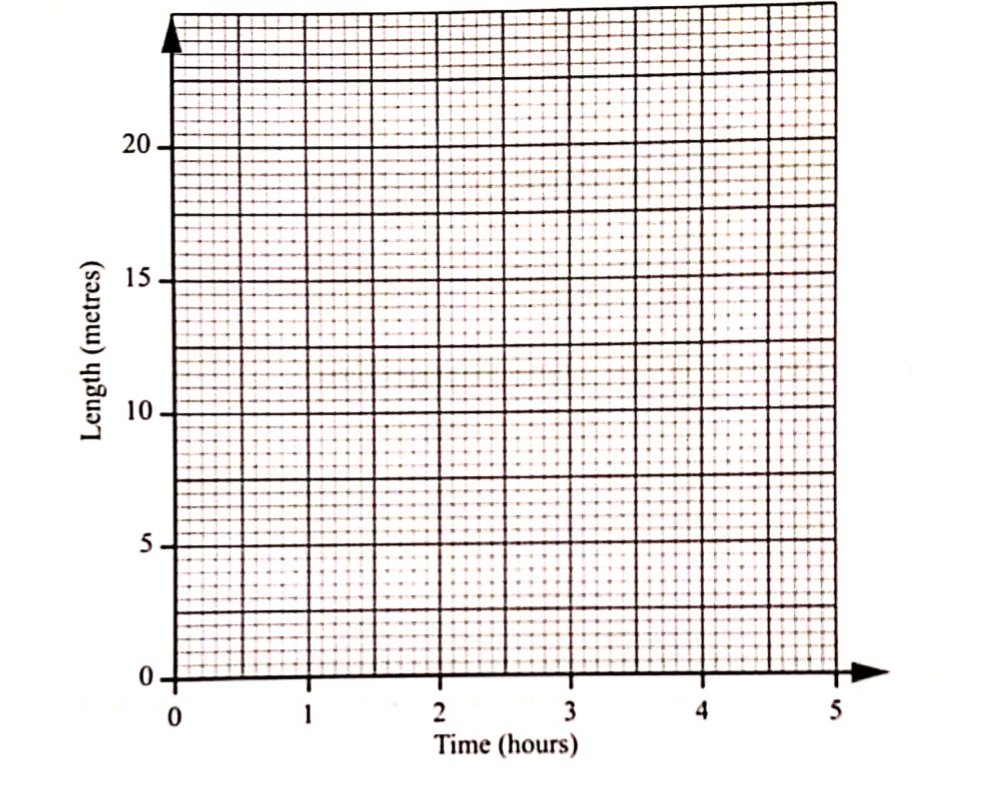
(b) Dctermine the rate of change of the shadow’ length at = 2 (2 marks)

SECTION II (50 MARKS)

Answer any five questions

17.The first term of an Arthmetic Progression(AP) is equal to the first term of a Geometric Progression (GP). The second team of the AP is equal to the fourth term Of the GP while the tenth term of the AP is equal to the seventh term of the GP.

(a) Given that a is the first term and d is the common difference of the AP while r is the common ratio of the GP, write the two equations connecting the AP and the GP. (2 marks)

(b) Find the value of r that satisfies the progressions.(4 marks)

(c) Given that the tenth term of the GP is 5120, find the values of a and d.(2 marks)

(d) Calculate the sum of the first 20 terms of the AP.( 2 marks)

18. Mbaka bought some plots at Ksh 400000 each. The value of each plot appreciated at the rate of 10% per annum. (a) Calculate the value of a plot after 2 years. (2 marks)

(b) After some time t, the value of a plot was Ksh 558400. Find i, to the nearest month. (4 marks)

(c) Mbaka sold all the plots he had bought after 4 years for Ksh2 928200.

Find the percentage profit Mbaka made, correct to 2 decimal places. (4 marks)

19. The figure KLMN below is a scale drawing of a rectangular piece of land of length KL = 80m

 

(a) On the figure, construct

(i) The locus of a poini P which is both equidistant trom points L and M It and from lines KL and LM. (3 marks)

(ii) the locus of a point Q such that ∠KQL = 90°. (3 marks)

(b) (i) Shade the region R bounded by the locus of Q and th Locus of poinis equidistant from KL and LM. (3 marks)

(ii) Find the area of the region R in m². (Take ℼ= 3.142). (3 marks)

20. A ship left point P(l0°S, 40°E) and sailed due East for 90 hours at an average speed of 24 knotS to a point R.

(Take I nautical mile (nm) to be 1.853 km and radius of the earth to be 6370 km) (a) Calculate the distance between P and R in:

(i) nm; (1 marks)

(ii) km. (1 marks)

(b) Determine the position of point R.( 5 marks)

(c) Find the local time, to the nearest minute, at point R when the time at P is II:00a.m. (3 marks)

21. A workshop makes cupboards and tables using two artisans A and B every cupboard made requires 3 days of work by artisan A and 2 days of work by artisan B.

Every table made requires 2 days of work by artisan A and 2 days of work by artisan B.

In one month artisan A worked in less than 24 while artisan B Worked for Not More Than 18 Days.

The workshop made x cupboards and y tables in that month.

(a) Write all the inequalities which must be satisfied by x and y. (3 marks)

(b) Represent the inequaliiies in (a) on the grid provided.(3 marks)

 

(c) The workshop makes a profit Of Ksh 6 000 on each cupboard and Ksh4 000 on each table.

Find the number of cupboards and the number of tables that must be made for maximum profit and hence determine the maximum profit. (4 marks)

22. The amount of money contributed by a group of students during a fundraising for a needy student was as shown in the table below.

 

Amount(  Ksh)	301 – 400	401 – 500	501 – 600	601 – 700	701 – 800	801 – 900	901 – 1000
No. of students	2	10	12	14	7	3	2

(a) On the grid provided draw an ogive to represent the data. (4 marks)

(b) Use the graph to estimate:

(i) The median; (1 marks)

(ii) The quartile deviation; (3 marks)

(iii) The percentage number of students who contributed at least Ksh 750.50. (2 marks)

23. In the figure below, OA = a,OB = b and BX meets OY at C.OX:OA = 1:2 and BY:YA = 1:3.

 

(a) Express in terms of a and b: (1 marks)

(ii) OY; (2 marks)

(iii) BX. (1 marks)

(b) Given that OC = hOY and BC = kBX, determine the values of h and k. (6 marks)

24. A trapezium PQRS with vertices P(2, 2), Q(6, 2), R(6, 4) and S(2, 8) is mapped onto P’Q’R’5′ by a transformation matrix M = (—1/0 0/1).

(a) Find the coordinates of P’Q’R’ S’.(2 marks)

(b) On the grid provided draw PQRS and its image P’Q’R’S’ (2 marks)

 

(c) Find P”Q”R”S“, the image of P’Q’R’S’ under the transformation matrix. N = (-½/0 0/-½)(1 marks)

(ii) On the same Grid draw P”Q”R‘S”.(1 marks)

(d) (i) Find a single matrix that maps P”Q”R’S” onto P’Q’R’S’.(2 marks)

(ii) Describe fully the transformation thai maps P”Q”R”S” onto P’Q’R’s’.(2 marks)

 

Questions and Answers

2019 Mathematics Paper 2