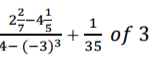KNEC KCSE Mathematics Paper 1 Question Paper / 2015 KCSE Starehe Boys Centre Mock
2015 KCSE Starehe Boys Centre Mock
Mathematics Paper 1
SECTION A (50 Marks)
Answer ALL questions from this section
Without using calculators evaluate 1/3 of (2¾ – 5½) x 36/7 ÷ 9/4
2 marks
Use the method of completing the square to solve the quadratic equation
2×2 – 13x + 15 = 0
3 marks
Solve for θ in the equation 6cos2 θ – Sin θ – 4 = 0 in the range 0o < θ < 180o.
3 marks
The sides of a rectangle are x cm and (x + 1) cm. A circle has radius of (x + 2) cm. If the sum of the area of the rectangle and the circle is 184 cm2. Using π as 227 find the value of x.
4 marks
Use binomial expansion to evaluate 2+12+2-12
3 marks
A line L1 passes through point (1, 2) and has a gradient of 5. Another line L2 is perpendicular to L1 and meets it at a point where x = 4. Find the equation for L2 in the form y = mx + c.
4 marks
Find the value of x in the following equation.
9x+32x-1=53
3 marks
The first and the last terms of an AP are 2 and 59 respectively. If the sum of the series is 610, find the number of terms in the series and the common difference.
4 marks
The equation of a circle is 2×2+2y2+12x-20y-4=0 . Determine the coordinates of the centre of the circle and state its radius.
3 marks
Make b the subject of the formula a=bdb2-d
3 marks
Solve the inequality 3-2x≤x≤2x+53 and show the solution on the number line.
4 marks
Solve for x given that log25x-log42x=3
3 marks
A salesman earns a basic salary of sh. 9,000 per month. In addition he is also paid a commission of 5% for sales above sh. 15,000. In a certain month he sold goods worth sh. 120,000 at a discount of 2½%. Calculate his total earnings that month.
3 marks
A small cone of height 8 cm is cut off from a bigger cone to leave a frustum of height 16 cm. If the volume of the smaller cone is 160 cm3, find the volume of the frustum.
3 marks
Vector OP = 6i + j and OQ = -2i + 5j. A point N divides PQ internally in the ratio 3:1. Find PN in terms of i and j.
3 marks
Without using mathematical tables or calculators express in surd form and simplify
1+cos30o1-sin60o
3 marks
SECTION B (50 Marks)
Answer any five questions in this section
In the figure below, vector OP = p and OR = r. OS = 2r and OQ : OP = 3 : 2
(a) Express the following vectors in terms of p and r.
(i) QR
(ii) PS
(b) The lines QR and PS intersect at K. By expressing OK in two different ways, find the ratio PK : KS
10 marks
On the graph paper provided, plot the triangle
(a) whose co-ordinates are A(1, 2) B(5, 4) and C(2, 6) {1 mark}
(b) On the same axes
(i) Draw the image A1B1C1 of ABC under a rotation of 90o clockwise about origin. {2 marks}
(ii) Draw the image A11B11C11 of A1B1C1 under a reflection in the line y = -x. State the
coordinates of A11B11C11. {3 marks}
(c)A111B111C111 is the image of A11B11C11 under the reflection in the line x = 0. Draw the image A111B111C111 and state its coordinates. {2 marks}
(d) Describe a single transformation that mapsA111B111C111 onto ABC.
10 marks
A bus left Kitale at 10.45 a.m and travelled towards Nairobi at an average speed of 60 km/h. A Nissan left Kitale on the same day at 1.15 p.m and travelled along the same road at an average speed of 100 km/h. The distance between Kitale and Nairobi is 500 km.
(a) Determine the time of the day when the Nissan overtook the bus. {6 marks}
(b) Both vehicles continued towards Nairobi at their original speed. Find how long the Nissan had to wait in Nairobi before the bus arrived. {4 marks}
10 marks
The table below shows how income tax was charged in a certain year.
| (Kenya pounds) | (Ksh. per Kenya pound) |
| 1 – 3630 | 2 |
| 3631 – 7260 | 3 |
| 7261 – 10890 | 4 |
| 10891 – 14520 | 5 |
| 14521 – 18150 | 6 |
| 18151 – 21780 | 7 |
| 21781 and above | 7.5 |
During the year Mwadime earned a basic salary of Ksh. 25,200 and a house allowance of Ksh. 12,600 per month. He was entitled to a personal tax relief of Ksh. 1,162 per month.
(a) Calculate:
(i) Mwadime’s taxable income in Kenya pounds per annum. {2 marks}
(ii) The net tax he pays per month. {6 marks}
(b) Apart from income tax he also contributes monthly NHIF Ksh. 1600, WCPS Ksh. 1000. Calculate his net monthly pay. {2 marks}
10 marks
X, Y and Z are three quantities such that X varies directly as the square of Y and inversely as the square root of Z.
(a) Given that X = 18 when Y = 3 and Z = 4, find X when Y = 6 and Z = 16. {5 marks}
(b) If Y increases by 10% and Z decreases by 19%, find the percentage increase in X. {5 marks}
10 marks
(a) A port B is on a bearing 080o from a port A and a distance of 95 km. A Submarine is stationed at a port D, which is on a bearing of 200o from A, and a distance of 124 km from B. A ship leaves B and moves directly Southwards to an Island P, which is on a bearing of 140o from A. The Submarine at D on realizing that the ship was heading to the Island P, decides to head straight for the Island to intercept the ship. Using a scale of 1 cm to represent 10 km, make a scale drawing showing the relative positions of A, B, D and P. {4 marks}
Hence find:
(b) The distance from A to D. {2 marks}
(c) The bearing o the Submarine from the ship when the ship was setting off from B. {1 mark}
(d) The bearing of the Island P from D. {1 mark}
(e) The distance the Submarine had to cover to reach the Island P. {2 marks}
10 marks
The data below represent the heights taken to the nearest centimeters of 40 lemon trees in a garden. (NB: A = Assumed mean)
| Height (cm) | f | X | d = x – A | fd | d2 | fd2 |
| 131 – 140 | 3 | |||||
| 141 – 150 | 4 | |||||
| 151 – 160 | 7 | |||||
| 161 – 170 | 11 | |||||
| 171 – 180 | 9 | |||||
| 181 – 190 | 5 | |||||
| 191 – 200 | 1 |
(a) Complete the table. {6 marks}
(b) Using 165.5 as the assumed mean, calculate the mean height. {2 marks}
(c) Calculate the standard deviation of the distribution. {2 marks}
10 marks
The line segment BC = 7.5 cm long is one side of triangle ABC.
(a) Use a ruler and compasses only to complete the construction of triangle ABC in which
∠ABC = 45o, AC = 5.6 cm and angle BAC is obtuse. {3 marks}
(b) Draw the locus of a point P such that P is equidistant from a point O and passes through the vertices of triangle ABC. {3 marks}
(c) Locate point D on the locus of P equidistant from lines BC and BO. Q lies in the region enclosed by lines BD, BO extended and the locus of P. Shade the locus of Q. {4 marks}