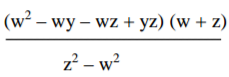KNEC KCSE Mathematics Paper 1 Question Paper / 2016 KCSE 4MCK Joint Exam
2016 KCSE 4MCK Joint Exam
Mathematics Paper 1
SECTION I (50 Marks)
Answer all the questions in this section in the spaces provided.
If 1⋅2 = 𝑎a/b , find the value of 2a – √b
3 marks
a) Using squares, square roots and reciprocal tables only, find the value of
i) 0⋅0596 (1 mark)
ii) ( 1/4⋅327)2 (1 mark)
b) Hence, find the value of
(0⋅0596)½ + ( 1/4⋅327)2 (2 marks)
4 marks
Find the value of k, if
3 marks
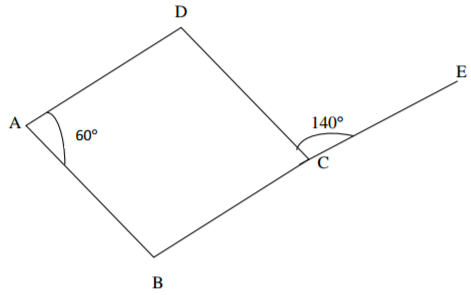
In the figure below, ABCD is a kite in which BC is produced to E. <DCE = 140° and
<BAD = 60°.
Calculate the size of
a) <ABC (2 marks)
b) <BCA (1 mark)
3 marks
Simplify the expression
3 marks
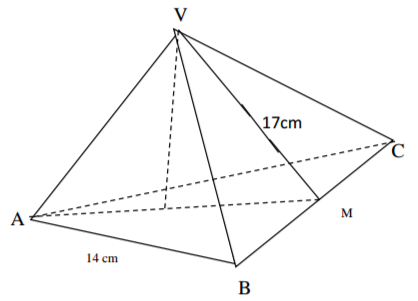
The figure below is a solid pyramid. The base is an equilateral triangle of side 14cm.
Point V is vertically above O and M is the midpoint of BC. VM is 17cm.
If the solid weights 0⋅293kg, calculate its density in g/cm3
3 marks
Mutua sold a car to Kyalo at a loss of 20%. Kyalo sold the car to Muema at a profit of
50%. Muema sold the car to Musyoka at a l,oss of 25%. Calculate the percentage profit
Kyalo would have made, had he sold the car directly to Musyoka.
3 marks
Find the image of point A (6,-2) under the transformation defined by
X′ = x – 3y
Y′ = 2x
2 marks
An inverted cone has a height of 72cm and a base radius of 49cm contains water to a
depth of 28cm. Calculate the volume of water in liters, refined to fill up the cone.
3 marks
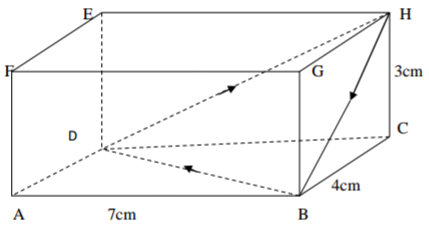
A rectangular prism ABCDEFGH has a tought string tied from H to B to D and back to it
as shown below
a) Draw the net of the prism and label. (2 marks)
b) On the net, show the string, and measure its length. (2 marks)
4 marks
A bus can carry a maximum of 61 passengers when full. The bus has a total of 24 seats,
some of which can accommodate 2 passengers and other 3 passengers. Determine the
number of seats in the bus.
2 marks
Two pipes fill a swimming bath in 18 hours. The samller pipe takes 10½ hours longer
than the larger pipe to fill the bath. How long does the longer pipe take to fill the bath?
4 marks
The perimeter of a quadrant of a circle is 137⋅5cm. Find its area.
3 marks
The length of certain plants in cm, were recorded as follows
| Length (cm) | 11 – 20 | 21 – 30 | 31 – 40 | 41 – 50 | 51 – 60 |
| Frequency(f) | | | | | |
| Cumulative frequency | 4 | 12 | 28 | 48 | 58 |
a) Complete the table. (2 marks)
b) Draw a frequency polygon to represent the above data. (3 marks)
5 marks
Simplify
1/Cos2 x – Tan2x
2 marks
The figure below is a map of a forest drawn on a grid of 1cm squares.
a) Estimate the area of the map in cm2 (1 mark)
b) If the scale of the map is 1:50,000 estimate the area of the forest in hectares. (2 marks)
3 marks
SECTION II (50 Marks)
Answer only five question in this section in the spaces provided.
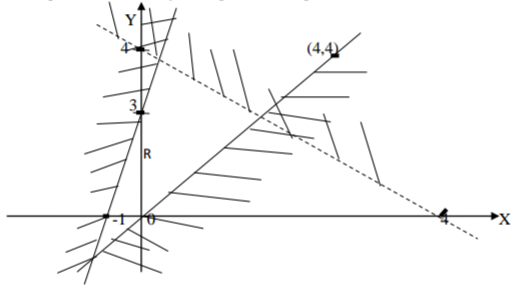
a) Form three inequalities that satisfy the region in the figure below. (3 marks)
b)Solve the following inequalities and represent your answer on a number line
7x – 4 ≤ 9x + 2 < 3x + 14 (3 marks)
c) Find the distance of the point (4,3) from the point of intersection of the lines 2x – 3y = 7
and 4x + 3y = 5, leaving your answer in simplified surd form. (4 marks)
10 marks
Musyoka left town A at 8:00am and travelled towards town B at an average speed of
64km/hr. At 8:30am, John from town B and travelled towards town A at the same speed.
The two towns are 384km apart.
a) At what time of the day did they meet? (5 marks)
b) How far from B was their meeting point? (2 marks)
c) How far apart were they at 10:30am? (3 marks
10 marks
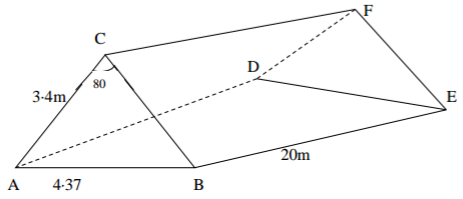
The diagram below represents a metallic frame for marking a children’s shelter in a
refugee camp. The ends are triangular such that AB = DE= 4⋅37m, BC = AC = 3⋅4m. CF
= AB = BE = 20m. <ACB = 80°
a) Calculate to 1 decimal place the vertical height of the tent. (2 marks)
b) Find the area to 1 decimal place of the canvas material needed to cover the tent
completely excluding the floor area. (3 marks)
c) If the cost of 1m of the metal used is Kshs 300, and the cost per square meter of the
canvas is Kshs 180. Calculate the cost of materials used to make three such tents.
(5 marks)
10 marks
Jane is standing 10km from a point P, which is due North of her. She walks on a straight
path on a bearing of 030°.
a) How far will she have walked when she is equidistant from the starting point and the
point P. (2 marks)
b) find the bearing of P from this equidistant point, to the nearest degree (4 marks)
c) How far will Jane have walked when she is at the shortest distance from the point P?
(2 marks)
d) How far will the point P be, from Jane when she has walked 20km on the same straight
path, to 2 decimal placeS. (2 marks)
10 marks
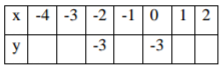
a) Complete the table below for the function y = 2×2 + 4x – 3 (2 marks)
b)Using the values in the completed table draw the graph of y = 2×2 + 4x – 3 (3 marks)
Scale: 2cm ≡ 1 unit (x); 2cm ≡ 1 unit (y)
c) Using your graph, estimate the roots of the equation
X2 + 2x – 1⋅5 = 0 to 1 decimal place (2 marks)
d) By drawing a suitable straight line on the same axes, solve the equation. (3 marks)
x2 + x – 5 = 0, to 1 d.p
10 marks
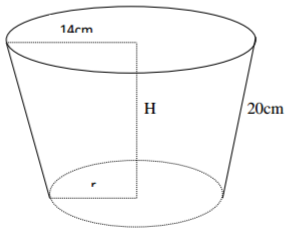
The pail shown below is open at the top and is in the shape of a frustram of a cone. The
pail is filled with water to the brim, and the total surface area of the surface in contact
with water is 380πcm2. The slant height of the pail is 20cm. The radius of the top circular
part is 14cm, and the circular bottom is r cm.
Calculate the
a) Base radius r, in cm (4 marks)
b) Height, h, of the pail in cm (2 marks)
c) Volume of water in the pail, in liters, in terms of π (4 marks)
10 marks
Points A, B, and C have the co-ordinates (3,1), (8,2) and (2,6) respectively.
a) Find the midpoints of (2 marks)
i) AB
ii) AC
b) Determine the equations of the perpendicular bisectors of (4 marks)
i) AB
ii) AC
c) Hence determine the area of the circle which passes through the points A, B, and C,
leaving your answer in terms of π. (4 marks)
10 marks
The displacement, h meters, of a particle moving along a straight line after t seconds is
given by h = -2t3 + 3/2t2 + 3t
a) Find it is initial acceleration (3 marks)
b) Calculate
i) The time when the particle is momentarily at rest (3 marks)
ii) Its displacement by the time it comes to rest momentarily. (2 marks)
c) Calculate the maximum speed attained. (2 marks)
10 marks