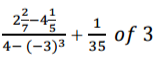KCSE Mathematics Paper 1 – 2015 KCSE Ikutha Sub-County Joint Examination
2015 KCSE Ikutha Sub-County Joint Examination
Mathematics Paper 1
SECTION I (50 Marks)
Answer all the questions in this section.
Without using mathematical tables or a calculator, evaluate
2 marks
Solve the equation 9x + 32x = 486.
3 marks
A sales man earns a basic salary of sh. 9000 p.m. In addition he is also paid a commission of 5% for
sales above sh.15,000. In a certain month he sold goods worth sh.120,000 at a discount of 2½%.
Calculate his total earning that month.
3 marks
Each interior angle of a regular polygon is 120o larger than the exterior angle. How many sides does the polygon has?
3 marks
Given the equation 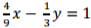
Leave your answer in the form y = mx + c.
3 marks
Solve for θ in the trigonometric equation given by 4 – 6 Cos2 θ = Sin θ for 0o ≤ 𝜃θ ≤ 360o.
4 marks
Given that A is the point (-8, 4) and B is the point (-12, -12), find the coordinates of a point K on AB
such that :
a) AK:KB = 2:3 (2 marks)
b) AK:KB = 5: -1 (2 marks)
4 marks
Two fair dice are tossed and the outcome on each dice is recorded. Find the probability that the sum on both dice is greater than or equal to 7.
3 marks
Express in surd form and simplify by rationalizing the denominator.
3 marks
Solve
2x – 5y = 1
4x2 + 25y2 = 41
3 marks
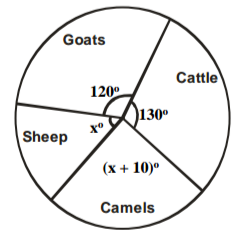
A farmer has four types of animals on his farm. The pie chart below represents the number of animals on the farm. If the number of goats were 30, calculate the number of camels on the farm.
4 marks
Find the value of x for which 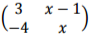
2 marks
A water tank has a capacity of 70litres. A similar model tank has a capacity of 0.25litres. If the larger
tank has a height of 150cm. Calculate the height of the model tank.
3 marks
The sides of a rectangle are given as 4.2cm and 2.8cm, each correct to one decimal place. Find the
maximum percentage error in its area.
3 marks
Find the equation of a circle whose centre lies on the line 3y = 6x + 18 and which also touches the x and y axes in the third quadrant.
4 marks
In the figure below O is the centre of the circle ABCD and AOD is a straight line. If AB =BC and the
angle DAC = 40o
Calculate angle BAC.
3 marks
SECTION II (50 Marks)
Answer any FIVE questions in this section.
Tickets for a football match cost 100 shillings and 50 shillings and tickets to the value of Ksh.100,000
were sold. If 30% more tickets of sh.50 and 40% fewer tickets of sh.100 had been sold, the income
would have increased to Ksh.112, 500. How many tickets of each category were sold?
10 marks
Given that A(-7, 2), B(-3, 3), C(-3, 6), D(-7, 8) are the vertices of a trapezium.
a) Draw the trapezium on the grid provided. (1 mark)
b) Rotate ABCD through -900
about the origin to be mapped onto A1B1C1D1. (2 marks)
c) Reflect A1B1C1D1 along y = -x to be mapped onto A11B11C11D11. (2 marks)
d) A certain transformation maps A11B11C11D11 to A111 (-7, -9) B111 (-3, -6) C111 (-3, -9) D111 (-7, -15).
i) Plot A111B111C111D111 on the same axis. (1 mark)

iii) Find the area of A111B111C111D111 (1 mark)
10 marks
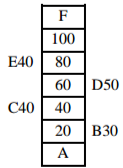
The field book below gives measurement of a field. The distances are given in metres. AF= 100m.
a) Using a scale of 1cm represents 10m draw a map of the field with straight boundary edges. (4 marks)
b) i) Find the area of the field in square metres. (5 marks)
ii) Determine the area of the field in hectares. (1 mark)
10 marks
Use a ruler and compass only for all the constructions in this question.
a) Construct a triangle XYZ in which XY= 6cm, YZ =5cm and angle XYZ= 120o. (2 marks)
b) Measure XZ and angle YXZ. (2 marks)
c) Construct the perpendicular bisector of XZ and let it meet XZ at N. (1 mark)
d) Locate a point W on the opposite of XZ as Y and that XW = ZW and YW =9cm and hence complete
triangle XZW. (2 marks)
e) Measure WM and hence calculate the area of triangle XZW. (3 marks)
10 marks
The table below shows the masses of newly born babies at a maternity home.
a) Complete the table and use it to answer the questions below. Take A = 3.7 (6 marks)
b) Use the method of assumed mean to calculate to two decimal places.
i) The mean mass of the babies. (2 marks)
ii) The standard deviation of the distribution. (2 marks)
10 marks
a) Complete the table below, for the function y =2x2 + 4x – 3. (2 marks)
b) On the grid provided, draw the graph of y=2x2 + 4x – 3 for -4≤ x≤ 2 and use the graph to estimate
the roots of the equation to 1 decimal place. (4 marks)
c) In order to solve graphically 2x2 + x – 5 = 0, a straight line must be drawn to intersect the curve
y = 2x2 + 4x – 3.
Determine the equation of the straight line and draw it, hence obtain the roots of the equation
2x2 + x – 5 = 0 to 1 decimal place. (4 marks)
10 marks
a) A carpet measuring (x+4)m by (x-1)m laid down in a rectangular room measuring 2x m by x m
leaving out uncovered floor near the walls round the room. If the carpet is 36m2 , calculate the area
of the uncarpeted floor. (6 marks)
b) If 20cm square tiles were to be used to carpet the uncarpeted section of the floor in (a) above,
calculate the cost of carpeting the whole floor if the carpet costs sh.300 per square metre and each
tile costs sh.100 per square metre. (4 marks)
10 marks
Mwikali is standing at a point P, 160m South of a hill H on a level ground. From point P she observes
the angle of elevation of the top of the hill to be 67o.
a) Calculate the height of the hill. (3 marks)
b) After walking 420m due East to the point Q, Mwikali proceeds to point R due East of Q where the angle of elevation of the top of the hill is 35o . Calculate the angle of elevation of the top of the hill from Q.(3 marks)
c) Calculate the distance from P to R. (4 marks)
10 marks