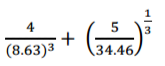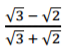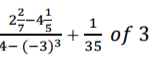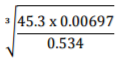KCSE Mathematics Paper 2 – 2015 KCSE Ikutha Sub-County Joint Examination

Use tables of cubes, cube roots and reciprocals to find the value of;

Make x𝑥 the subject of the formula

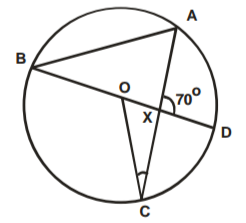
In the figure below, BOD is the diameter of the circle centre O. Angle ABD = 30^o and angle AXD=70^o‍

Determine the size of:
a) Reflex angle BOC. (2 marks)
b) Angle ACO. (1 mark)

Solve for x in the equation
27^{x – 1} × 3^{x + 1} = 243

Simplify the expression below in the form of a + b√𝑐c

Find the value of x that satisfies the equation log₂ (x +5) = 2 – log₂ (x +2)

A variable P varies jointly with the square of R and inversely with the square root of Q. If R is increased by 10% and Q decreased by 20%, what is the percentage change in the value of P.

Three grades of tea A, B and C costing sh.280, sh.190 and sh.170 per kg respectively are mixed in the
ratio 3:4:K. Find the value of K for which when the mixture is sold at sh.250 per kg, 25% profit is
realized.

Expand (1 – 2x)⁵ hence use the expansion up to the forth term to estimate the value of (0.8)⁵ correct to 3 decimal places.

Find the value of x such that 8 Cos²x – 2 Cos x – 1 = 0, for 0^o ≤ x ≤ 360^o.

Find the equation of the normal to the curve y = 3x² – 9x at the point (2, -6). Express your answer in the form y = mx + c.

The figure below shows a circle in which chord AD and BC intersect at G. AB and CD extended meet
at K. Given that KB= 5cm, KC=15cm and DK= 7cm, determine the length of KA.

If a man deposited Ksh.2000 in a bank which pays 8% interest per annum compounded quarterly, how long will it take until there is Ksh.3000 in the bank? (Leave your answer correct to 1 decimal place.

A clearing company has two machines M₁ and M₂. Both machines working together can complete a
piece of work in 6 hours while M₁ working alone can complete the work in 15 hours. M₂ worked alone for 4 hours before M₁ was switched on and the two of them worked together. Find the time taken by M₁ and M₂ to complete the remaining work.

In a drama committee of 90 members, the ratio of women to men is 2:7. Find the number of women
required to join the committee so that the ratio of men to women is 5:4.

Given that the expression 4y² – 8y + 3p + 13 is a perfect square,

a) Find the value of P. (2 marks)

b) Hence solve the equation
4y² – 8y + 3p + 13 = 0. (2 marks)

a) The product of the third and the second of an arithmetic sequence is 0. If the common difference is 10, find the first term. (3 marks)

b) In a geometric progression, the sum of the second and the third is 4 and the sum of the third and the fourth term is 2. Find the first term and the common ratio. (4 marks)

c) The sum of the first 8 terms of an AP is 220. If the third term is 17, find the first term and the
common difference. (3 marks)

Two towns on the earth’s surface are located at P(07^o N, 30^o E) and Q(13^o S, 30^o E). A pilot plans to fly from P to Q by the shortest route between the two towns.

a) Calculate the shortest distance between P and Q in km. (5 marks)

b) Find this distance in nautical miles (nm). (3 marks)

c) The speed of the aircraft is 360 knots. Determine how long it takes to fly from P to Q. (2 marks)

Mutua has three sets of balls to play table tennis with. He has 4 yellow balls, 6 blue balls and 2 white
balls, all of the same size and shape. He picks a ball at random without replacement and its colour
noted. If he picks a yellow ball the probability of winning table tennis is 1/3. If he picks a blue ball the
probability of winning is 1/4 otherwise with a white ball the probability of winning is 5/6.

a) If he is allowed to pick 2 balls one at a time, determine the probability that at least one of the balls
picked is blue. (4 marks)

b) Find the probability of Mutua picking a white ball and lose the game having picked once. (1 mark)

c) Find the probability of Mutua winning the game with either a white or blue ball picked twice, one at a time. (2 marks)

d) A ball was picked with replacement. Determine the probability that at least one of the first two balls
picked was yellow and the game was won. (3 marks)

The gradient function of a curve is given by the expression 2x + 1. If the curve passes through the point (-4, 6)
a) Find:
i) The equation of the curve. (3 marks)

ii) The value of x at which the curve cuts the x-axis. (3 marks)

b) Determine the area enclosed by the curve and the x-axis. (4 marks)

An institute offers two types of courses, veterinary and agriculture. The institute has a capacity of 500 students. There must be more agriculture students than veterinary students. At least 200 students must take veterinary courses.

Let x represent the number of veterinary students and y the number of agriculture students.

a) Write down three inequalities that describe the given conditions. (3 marks)

b) On the grid provided, draw the three inequalities. (3 marks)

c) If the institute makes a profit of Ksh.25,000 to train one veterinary student and Ksh.10,000 to train one agriculture student, determine;

i) The number of students that must be enrolled in each course to maximize the profit. (2 marks)

ii) The maximum profit. (2 marks)

x, y and z are three quantities such that x varies directly as the square of y and inversely as the square root of z.

a) Given that x =12, and y = 24, and z =36, find x when y = 27 and z = 121 (3 marks)

b) If y increases by 5% and z decreases by 19%, find the percentage increase in x. (5 marks)

c) If y is inversely proportional to the square root of x and that x =4 when y = 3, calculate the value of x when y = 8. (2 marks)

In the figure below, P, Q, R and S are points on the circle centre O. PRT and USTV are straight lines.
Line UV is a tangent to the circle at S. ∠RST = 50^o
and ∠RTV = 150^o‍

a) Calculate the size of;
i) Angle ORS (2 marks)
ii) Angle USP (1 mark)
iii) Angle PQR (2 marks)
b) Given that RT = 7cm and ST = 9cm, calculate to 3 significant figures;
i) The length of line PR. (2 marks)
ii) The radius of the circle. (3 marks)

A bus left Kitui and travelled towards Kibwezi at an average speed of 60km/h. After 2½ hours, a car left Kitui and travelled along the same road at an average speed of 100km/hr. If the distance between Kitui and Kibwezi is 500km, determine;

a) i) The distance of the bus from Kibwezi when the car took off. (2 marks)

ii) The distance the car travelled to catch up with the bus. (4 marks)

b) Immediately the car caught up with the bus, the car stopped for 25minutes. Find the new average
speed at which the car travelled in order to reach Kibwezi at the same time as the bus. (4 marks)