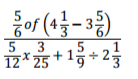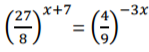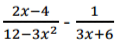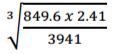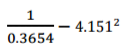KNEC KCSE Mathematics Paper 1 – 2015 – Machakos County Trial
2015 KCSE Machakos County Trial
Mathematics Paper 1
SECTION I (50 Marks)
Answer all the questions in this section in the spaces provided.
Evaluate without using a calculator.
2 marks
Without using a calculator or mathematical tables simplify.
3 marks
Find the value of x if
3 marks
Three sirens wail at intervals of thirty minutes, fifty minutes and thirty minutes. If they wail together at 7.18 a.m. on Monday, what time and day will they wail together?
3 marks
A two-digit number is such that the sum of the ones digit and the tens digit is 10. If the digits are
reversed, the number exceeds the original number by 54. Find the number.
3 marks
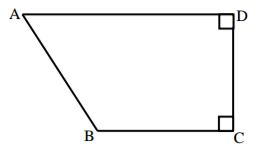
The figure below shows quadrilateral ABCD in which AB = 6cm. BC = ½CD, CD = DA and angle ADC
= angle BCD = 90o
Calculate the area of the quadrilateral ABCD. (4 Marks)
4 marks
The interior angle of a regular polygon is 108o larger than the exterior angle. How many sides has the
polygon?
3 marks
A salesman is paid a salary of Sh. 10,000 per month. He is also paid a commission on sales above Sh. 100,000. In one month he sold goods worth Sh. 500,000. If his total earning that month was Sh. 56,000.
Calculate the rate of commission. ( 3 Marks)
3 marks
A cylinder of radius 14cm contains water. A metal solid cone of base radius 7cm and height 18cm is
submerged into the water. Find the change in height of the water level in cylinder.
3 marks
Simplify the following.
3 marks
A mother is now 2½ times as old as her daughter Mary. Four years ago the ratio of their ages was 3:1.Find the present age of the mother.
3 marks
The line which joins the point A (3, k) and B (-2, 5) is parallel to the line whose equation is
5y + 2x – 7 = 0. Find the value of k.
3 marks
A Kenyan bank buys and sells foreign currencies at the exchange rates shown below.
| Currency | Buying (KShs.) |
Selling (KShs.) |
| 1 Euro 1 US Dollar |
147.86 74.22 |
148.00 74.50 |
An American arrived in Kenya with 20 000 Euros. He converted all the Euros to Kenya shillings at the
bank. He spent KShs. 2,512,000 while in Kenya and converted the remaining Kenya shillings into US
Dollars at the bank. Find the amount in Dollars that he received.
3 marks
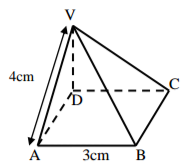
The diagram below represents a right pyramid on a square base of side 3cm. The slant edge of the
pyramid is 4cm.
(a) Draw a labelled net of the pyramid. (2 Marks)
(b) On the net drawn, measure the height of a triangular face from the top of the pyramid. (1 Mark)
3 marks
Using logarithms tables only, evaluate.
4 marks
Use reciprocal and square tables to evaluate, to 4 significant figures, the expression.
3 marks
SECTION II (50 Marks)
Answer only five questions in this section in the spaces provided.
A group of people planned to contribute equally towards buying land at a price of Shs 180,000. However 3 members of the group withdrew from the project. As a result, each of the remaining members were to contribute KShs. 3000 more.
(a) Find the original number of members in the group. (6 Marks)
(b) How much would each person have contributed if the 3 people had not withdrawn. (2 Marks)
(c) Calculate the percentage increase in the contribution per person caused by the withdrawal.(2 Marks)
10 marks
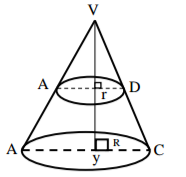
The figure below shows a cone from which a frustum is made. A plane parallel to the base cuts the cone two thirds way up the vertical height of the cone to form frustum ABCD. The top surface radius of the frustum is labelled r and the bottom radius R
(a) Find the ratio r:R. (1 Mark)
(b) Given that r = 7cm, find R. (2 Marks)
(c) If the height VY of the original cone is 45cm. Calculate to the nearest whole number the volume of
the frustum. (Take 𝜋π = 22/7) (4 Marks)
(d) The frustum represents a bucket which is used to fill a rectangular tank measuring 1.5m long, 1.2m wide and 80cm high with water. How many full buckets of water are required to fill the tank.
(3 Marks)
10 marks
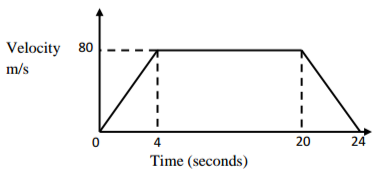
(a) The figure below is a velocity time graph for a car.
(i) Find the total distance travelled by the car. (2 Marks)
(ii) Calculate the deceleration of the car. (2 Marks)
(b) A car left Nairobi towards Eldoret at 7.12 a.m. at an average speed of 90km/h. At 8.22 a.m, a bus left Eldoret for Nairobi at an average speed of 72km/hr. The distance between the two towns is 348km.
Calculate:
(i) the time when the two vehicles met. (4 Marks)
(ii) the distance from Nairobi to the meeting place. (2 Marks)
10 marks
The following distribution shows the marks obtained by 82 students in a Mathematics test.
| Marks | 20-29 | 30-39 | 40-49 | 50-59 | 60-69 | 70-79 | 80-89 |
| Frequency | 3 | 18 | 13 | 14 | 17 | 12 | 5 |
(a) State the modal class. (1 Mark)
(b) Calculate to 2 decimal places:
(i) the mean mark (4 Marks)
(ii) the difference between the median and the mean marks. (5 Marks)
10 marks
John bought 3 brands of tea; A, B and C. The cost price of the three brands were Sh. 25, Sh. 30 and Sh. 45 per kilogram respectively. He mixed the three brands in the ratio 5:2:1 respectively. After selling the mixture, he made a profit of 20%.
(a) How much profit did he make per kilogram of the mixture? (4 Marks)
(b) After one year the cost price of each brand was increased by 10%.
(i) For how much did he sell one kilogram of the mixture to make a profit of 15%?
(Give your answer to the nearest 5 cents) (3 Marks)
(ii) What would have been his percentage profit if he sold one kilogram of the mixture at Sh. 45.
(3 Marks)
10 marks
Triangle PQR is inscribed in the circle. PQ = 7.8cm, PR = 6.6cm and QR = 5.9cm.
Find;
(a) size of angle QPR (3 Marks)
(b) the radius of the circle. (3 Marks)
(c) the area of the shaded region. (4 Marks)
10 marks
P, Q and R are three villages such that PQ = 10km, QR = 8km and PR = 4km are connecting roads.
(a) Using a scale of 1cm to represent 1km, locate the relative positions of the three villages. (2 Marks)
(b) A water tank T is to be located at a point equidistant from the three villages. By construction locate
water tank T and measure its distance from R. (2 Marks)
(c) Determine the shortest distance from T to the road PQ by construction. (2 Marks)
(d) Determine the area enclosed by the roads PQ, QR and PR by calculation. (3 Marks)
10 marks
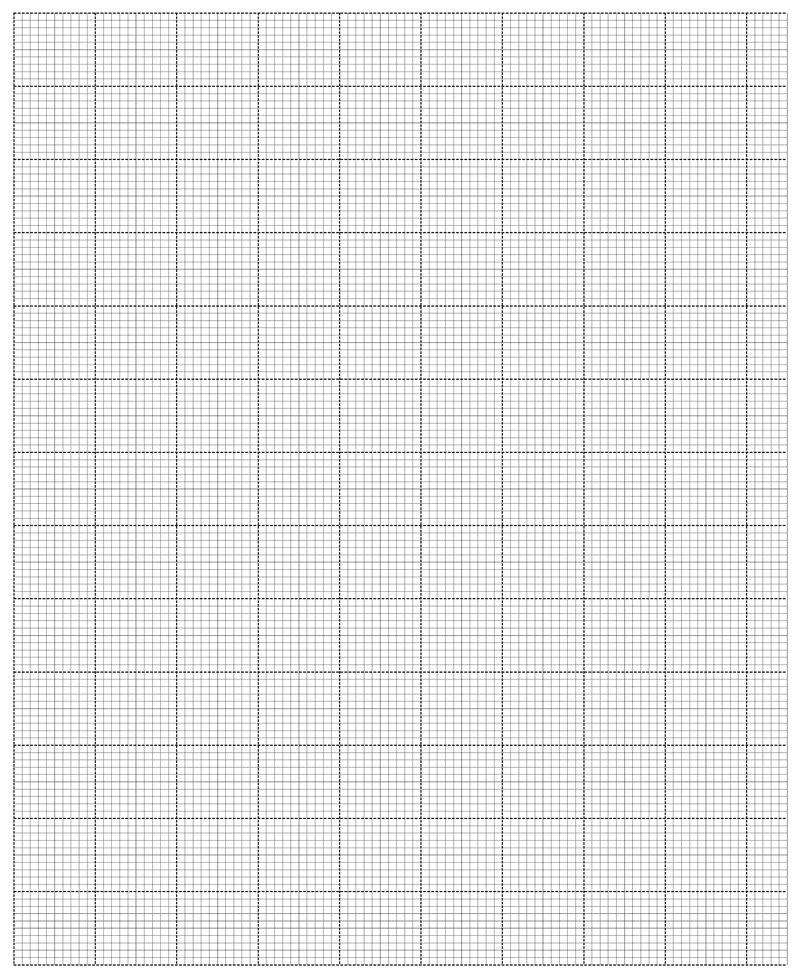
Triangle PQR has vertices at P (2,3), Q(1,2) and R(4,1), while triangle PIQIRI has vertices PI (-2,3),Ql (-1,2), RII(-4,1)
(a) (i) Draw triangle PQR and PIQIRI on the grid provided. (2 Marks)
(ii) Describe fully a single transformation which maps triangle PQR onto triangle PIQIRI. (1 Mark)
(b) (i) On the same grid, draw triangle PIIQIIRII the image of PQR under a reflection on the line y + x = 0
(2 Marks)
(ii) Describe fully a single transformation which maps triangle PIIQIIRII onto triangle PIQIRI. (1 Mark)
10 marks