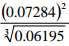KNEC KCSE Mathematics Paper 1 Question Paper / 2016 KCSE KAMDARA JET Examination
2016 KCSE KAMDARA JET Examination
Mathematics Paper 1
SECTION I (50 Marks)
Answer ALL the questions in this section
Without using a calculator or a mathematical table evaluate.
3 marks
By using substitution y = 3χ or otherwise solve, (4 marks)
3339 31 −=− xxx ++
3. Simplify: ( ) 124
2712 2
+−
−
x
x
3 marks
Simplify:
3 marks
A line L1 is perpendicular to the line 2x – 3y + 6 = 0. Find the angle made by line L1 and x axis.
3 marks
Three – fifths of a certain work is done on the first day. On the second day, 3/4 of the remainder
is completed. If on the third day 7/8 of what remained is done, what fraction of the work still
remains to be done?
3 marks
A bank in Kenya buys and sells foreign currency as shown in the table below.
Buying Selling
1 US dollar 100.87 100.97
1 Sterling pound 147.27 147.43
An American tourist came to Kenya with 15000 US dollars and converted the whole of it into
Ksh. He then spent Ksh. 650,000 and converted the remaining money to sterling pounds.
Calculate to the nearest pound the amount of money he remained with.
3 marks
Use logarithm tables to evaluate
4 marks
Under an enlargement scale factor -2, the image of A( 2 ,4) is A’(-1 ,-2). Under the same
enlargement, the image of D( x ,y) is D’(3, -2). Find the coordinates of the object D.
3 marks
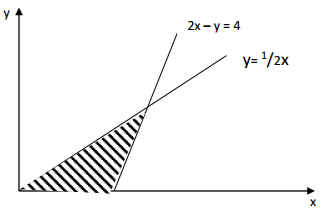
The figure below shows two lines 2x – y = 6 and y = ½ x, intersecting. Calculate the area of
shaded regions.
4 marks
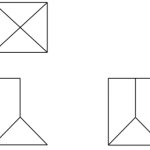
The diagram below represents a right pyramid on a square base of side 3cm. The slant edge of
the pyramid is 4cm.
(a) Draw a labeled net of the pyramid. (2 Marks)
(b) On the net drawn, measure the height of a triangular face from the top of the pyramid.(1 Mark)
3 marks
A salesman is paid a salary of Sh. 10,000 per month. He is also paid a commission on sales
above Sh. 100,000. In one month he sold goods worth Sh. 500,000. If his total earning that
month was Sh. 56,000. Calculate the rate of commission.
3 marks
Solve the following inequality and state the integral solutions.
( ) 424 x 2
1 − > ( ) ( ) 34236 xx 3
2
3
4 +−≥−
3 marks
A regular polygon is such that its exterior angle is one eighth the size of interior angle. Find the
number of sides of the polygon.
3 marks
The position vector of P is OP = 2i – 3j and M is the mid – point of PQ. Given OM = i + 4j,
Obtain the vector PQ.
3 marks
A liquid spray of mass 384 g is packed in a cylindrical container of internal radius 3.2 cm.
Given that the density of the liquid is 0.6g/cm3, calculate to 2 decimal places the height of liquid
in the container
3 marks
Given that sin (2ϴ + 30) = Cos (ϴ – 60). Find the value of tan ϴ to two decimal places.
2 marks
SECTION II (50 Marks)
Answer any FIVE questions only in this section
Water flows through a circular pipe of cross-sectional area of 6.16cm2 at a uniform speed of
10cm per second. At 6.00 a.m. water starts flowing through the pipe into an empty tank of base
area are 3m2
.
a) What will be the depth of the water at 8.30 a.m.? (5 marks)
b) If the tank is 1.2m high and a hole at the bottom through which water leaks at a rate of
11.6cm3 per second. Determine the time at which the tank will be filled. (5 marks)
10 marks
(a) The figure below is a velocity time graph for a car.
(i) Find the total distance travelled by the car. (2 marks)
(ii) Calculate the deceleration of the car. (2 marks)
(b) A car left Nairobi towards Eldoret at 7.12 a.m. at an average speed of 90km/h. At 8.22 a.m.,
a bus left Eldoret for Nairobi at an average speed of 72km/hr. The distance between the two
towns is 348km. Calculate:
i) the time when the two vehicles met. (4 marks)
ii) the distance from Nairobi to the meeting place. (2 marks)
10 marks
Using a ruler and a pair of compass only.
a) Construct a triangle ABC in which AB=8cm, BC=7.5 cm and ˂ABC = 112.50. (3 marks)
Measure length of AC. (1 mark)
b) By shading the required region show the locus of P within triangle ABC such that
i) AP ≤ BP
ii) AP˃3 (2 marks)
c) Construct a normal line from C to meet AB at D. (1 mark)
d) Locate the locus of R in the same diagram such that the area of the triangle ARB is 4
3 area
of triangle ABC. (3 marks)
10 marks
The diagram below represents a solid consisting of a hemispherical bottom and a conical
frustum at the top. O1O2=4cm, O2B=R=4.9cm O1A=r=2.1cm
a) Determine the height of the chopped off cone and hence the height of the bigger cone.
(2 marks)
b) Calculate the surface area of the solid. (4marks)
c) Calculate the volume of the solid. (4marks)
10 marks
a) Complete the table given below for the equation y = -2χ² + 3χ + 3 for the range -2 ≤ x ≤ 3.5
by filling in the blank spaces. (2 marks)
| x | -2 | -1.5 | -1 | -0.5 | 0 | 0.5 | 1 | 1.5 | 2 | 2.5 | 3 | 3.5 |
| y | | -6 | | 1 | | | | | | -2 | | -1 |
(b)Use the values from the table above to draw the graph of y = -2χ² + 3χ + 3. (3 marks)
(c)Use your graph to:
(i) Determine the integral values of χ in the graphs range which satisfy the inequality 2χ² – 3χ – 3 ≥ 3.
(3 marks)
(ii) Solve -2χ² + 2χ + 5 = 0. (2 marks)
10 marks
Triangle ABC has vertices A(3, 1), B(4, 4) and C(5, 2). The triangle is rotated through 900 about
(1, 1) to give A’B’C’. Triangle A’B’C’ is then reflected on the line y – x = 0 onto A’’B’’C’’.
triangle A’’B’’C’’ then undergoes enlargement scale factor – 1 through the origin to give
A’’’B’’’C’’’.
(a) On the graph paper, draw triangles A’B’C’, A’’B’’C’’ and A’’’B’’’C’’’. (8 marks)
(b) Describe the type of congruence between:
i) ΔABC and ΔA’B’C’
ii) ΔA’B’C’ and ΔA’’B’’C’’ (2 marks)
10 marks
The table below shows patients who attend a clinic in one week and were grouped by age as
shown in the table below.
| Age x years | 0≤ x < 5 | 5≤ x < 15 | 15≤ x < 25 | 25≤ x < 45 | 45≤ x < 75 |
| Number of patients | 14 | 41 | 59 | 70 | 15 |
(a) Estimate the mean age (4 marks)
(b) On the grid provided draw a histogram to represent the distribution. (3 marks)
(Use the scales: 1cm to represent 5 units on the horizontal axis 2 cm to represent 5unit on the
vertical axis)
(c) i) State the group in which the median mark lies ( 1 mark)
ii) A vertical line drawn through the median mark divides the total area of the histogram
into two equal. Using this information estimate the median mark. (2 marks)
10 marks
The figure below shows curve of y=2×2 + 4x + 3 and a straight line intersecting the curves at A
and B.
If the x – intercept is -3.5 and y – intercept as 7, find
a) The Equation of the straight line. (2 marks)
b) The coordinates of A and B. (4 marks)
c) The area of the shaded region. (4 marks)
10 marks