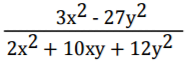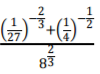KNEC KCSE Mathematics Paper 1 Question Paper / 2015 KCSE Kirinyaga West

A square of side (x + 2) cm has the same area as a rectangle measuring (2x + 4) cm and (x – 2) cm. Calculate the area of the rectangle.

Use the prime factors of 1936 and 1728 to evaluate.

Simplify the expression.

Two boats P and Q are located 45km apart, P being due north of Q. An observer at P spots a ship whose bearing he finds as S560. From Q the bearing of the same ship is N380E. Calculate the distance of the ship from Q to 2 decimal places.

The sum of interior angles of a regular polygon is 32400. Find the size of each exterior angle.

Simplify

Given that  and  evaluate ‍

Eight years ago the age of a father was six times the age of his son and after eight years from today the age of the father would be only twice the age of his son. Find their present ages.

The mass of a cylindrical metal rod of radius 14cm and height 10cm is 5.47kg. Find it’s density in g/cm³ to 2 decimal places.

Construct a DABC in which BC = 5cm, <B = 750 and <C = 600. From A drop a perpendicular to BC and measure its length to the nearest mm.

Find the values of x for which A has no inverse.

Solve 15 < 5 (3 – x) < 30 hence show your solution on a number line.

A major arc of a circle substends an angle of 2500 at the centre of a circle. If the radius of the circle is 9.8cm find the area of the minor sector. (Use π = 22/7).

A point A (-1, 3) is mapped onto A1(8, 12). Fidn the centre of enlargement given that the scale factor is 2.

A particle moving in a straight line has its displacement x metres from the origin O at time t seconds defined by the equation x = t³ – 6t² + 7. Determine the values of t for which the particle is momentarily at rest.

Maina can do a piece of work in 12 hours. Muthui can do it in 20 hours. How long would it take Muthui to complete the work if Maina has been working for 7 hours.

A line T passes through points (-3, -5) and (3, -6) and is perpendicular to a line l at (-2, -2).
a) Find the equation of l. (2 marks)
b) Find the equation of T in the form ax + by = c where a, b and c are constants. (2 marks)
c) Given that another line Q is parallel to T and passes through (1, -3) find x and y intercepts of Q. (3 marks)
d) Find the points of intersection of L and Q. (3 marks)

Use this velocity – time graph which represents the motion of a car for 10 seconds, to find:
a) The rate of acceleration. (1 mark)
b) The rate of retardation. (1 mark)
c) The total distance travelled. (2 marks)
d) The total distance travelled during the first 4 seconds. (2 marks)
e) The average speed maintained during this journey. (2 marks)
f) The distance travelled at the constant speed. (2 marks)

The percentage marks obtained by 40 students in a test are as under:
85, 30, 49, 62, 17, 84, 24, 15, 82, 61, 74, 38, 27, 13, 44, 72, 61, 49, 38, 23,
90, 32, 67, 18, 45, 58, 22, 46, 37, 39, 43, 55, 62, 30, 46, 59, 41, 26, 34 and 47.
a) Prepare a grouped frequency table from the above data using a class width of 10. (2 marks)
b) Use 49.5 as the working mean and estimate the mean from the grouped frequency table. (3marks)
c) Prepare a cumulative frequency table and draw the cumulative frequency curve on the grid of squares provided. (2 marks)
d) Use the cumulative frequency curve to estimate the median. (3 marks)

A calf runs in a straight line towards a cow with a velocity of vm/s after t seconds given by v = t (8 – t).
a) Complete the table below

(2 marks)
b) Hence draw the graph of v against t for 0 < t < 8 on the grid provided. (3 marks)
c) From the graph find the total distance the calf run.
i) Using eight trapezia of equal width. (3 marks)
ii) Using the exact method. (2 marks)

The figure below represents a game sanctuary in the shape of a quadrilateral in which AB = 30km,
AE = 20km and CE = 45km <BAC = 600, <EBC = 300 and <ECB = 17.100.

Calculate
a) The side BC correct to 2 decimal places. (3 marks)
b) The angle ABE to 1 decimal place. (2 marks)

c) The area of the game sanctuary in hectares correct to 2 decimal places. (3 marks)

Using a ruler and compasses only, construct a triangle ABC with AB = 4.5cm, <ABC = 75^o and <BAC = 60^o. Prolong CB and CA hence construct a circle that touches side AB and the prolonged sides. Calculate he area of the circle. Use p = 3.142.

The figure above shows a right pyramid VEFGHK. The base EFGHK is a regular pentagon. EO = 7cm and VE = 12cm.
Calculate:
a) The perimeter of the base to 2 decimal places. (3 marks)
b) The length VO to 2 decimal places. (1 mark)
c) The angle which edge VF makes with the edge FE. (3 marks)
d) The volume of the pyramid to 2 decimal places. (3 marks)

The equation of a curve is given by y = 2x³ + 3x² – 12x + 5.
a) Find the y – intercept of the curve. (1 mark)
b) Determine the stationery points of the curve. (4 marks)
c) Sketch the curve y = 2×3 + 3×2 -12x + 5 (5 marks)​